GOING IN CIRCLES [DAY 2]
EXPLORING PI: CIRCUMFERENCE & AREA OF CIRCLES
Leverage contextual problems to investigate and emerge Pi to help conceptualize formulas for both the circumference and area of a circle.
Intentionality
Spark Curiosity
Fuel Sensemaking
During Moves
Student Approaches
Next Moves
Consolidation
Reflect and Consolidation Prompts
Resources & Downloads
Educator Discussion Area
Intentionality & Unit Overview
Length of Unit: 8 Days
Access each lesson from this unit using the navigation links below
Students will engage in another exploration highlighting the relationship between circumference and diameter of a circle called Pi (π), then consolidate this new learning to emerge a formula for circumference of a circle.
Intentionality…
In this task, students will explore the concept of circumference through estimation followed by measurement. This task will allow students to develop a deeper understanding of big ideas, including the following:
- When exploring measurement relationships of any circle, there are three (3) standard measures that can be used to reveal more information about the circle:
- Circumference: the perimeter of a circle.
- Diameter: any straight line segment beginning and ending on the outer edge of a circle and passes through its centre.
- Radius: any straight line segment beginning at the centre of a circle and ending on the outer edge of the circle.
- Knowing one of the standard measures of a circle provides information about all other measures;
- There is a multiplicative relationship between the length of the diameter and the circumference of a circle. In other words, as the diameter of a circle increases, the circumference increases multiplicatively (not additively);
- For all circles, the ratio of the circumference to the diameter is the same (approximately 22:7); and,
- For all circles, the rate of each unit of circumference length per unit of diameter length is the same (approximately 22/7 or 3.14) and is known as Pi, π.
Note:
There are some very complex mathematical understandings happening “under the hood” when we explore the differences between ratios, rates, and other nuances along the journey through proportional relationships. If you want to build your mathematical content knowledge to assist in better understanding the progression through comparison, additive thinking, multiplicative thinking, ratios, rates, and proportional relationships, consider joining us in our 9-module self-paced course called The Concept Holding Your Students Back.
Lesson Walkthrough Video
Watch the following video that gives you a sneak peek into a virtual classroom where this lesson is being delivered via online learning:
Note that for privacy reasons, you cannot see student names or hear their voices, but you can get a sense as to how you might facilitate this lesson in a virtual environment and extrapolate to how this could look/sound in a face-to-face classroom environment.
Spark Curiosity
What Do You Notice? What Do You Wonder?
Show students the video below showing someone walking into a building a seeing a circle on the floor surrounded by white tiles.
Ask students to engage in a notice and wonder protocol. ANYTHING and EVERYTHING that comes to mind is fair game.
Write down all of the student noticing and wondering. For example:
- Is that a school?
- Who was holding the door?
- How big is the circle?
- Why is there a white spot on it?
- I noticed that there are square tiles around the outside.
- How many square tiles are there around the outer edge?
- And many others…
Take time to acknowledge the noticing and wondering your students have engaged in and try to answer any that you can address right away.
Estimation: Prompt
Then, land on the following question:
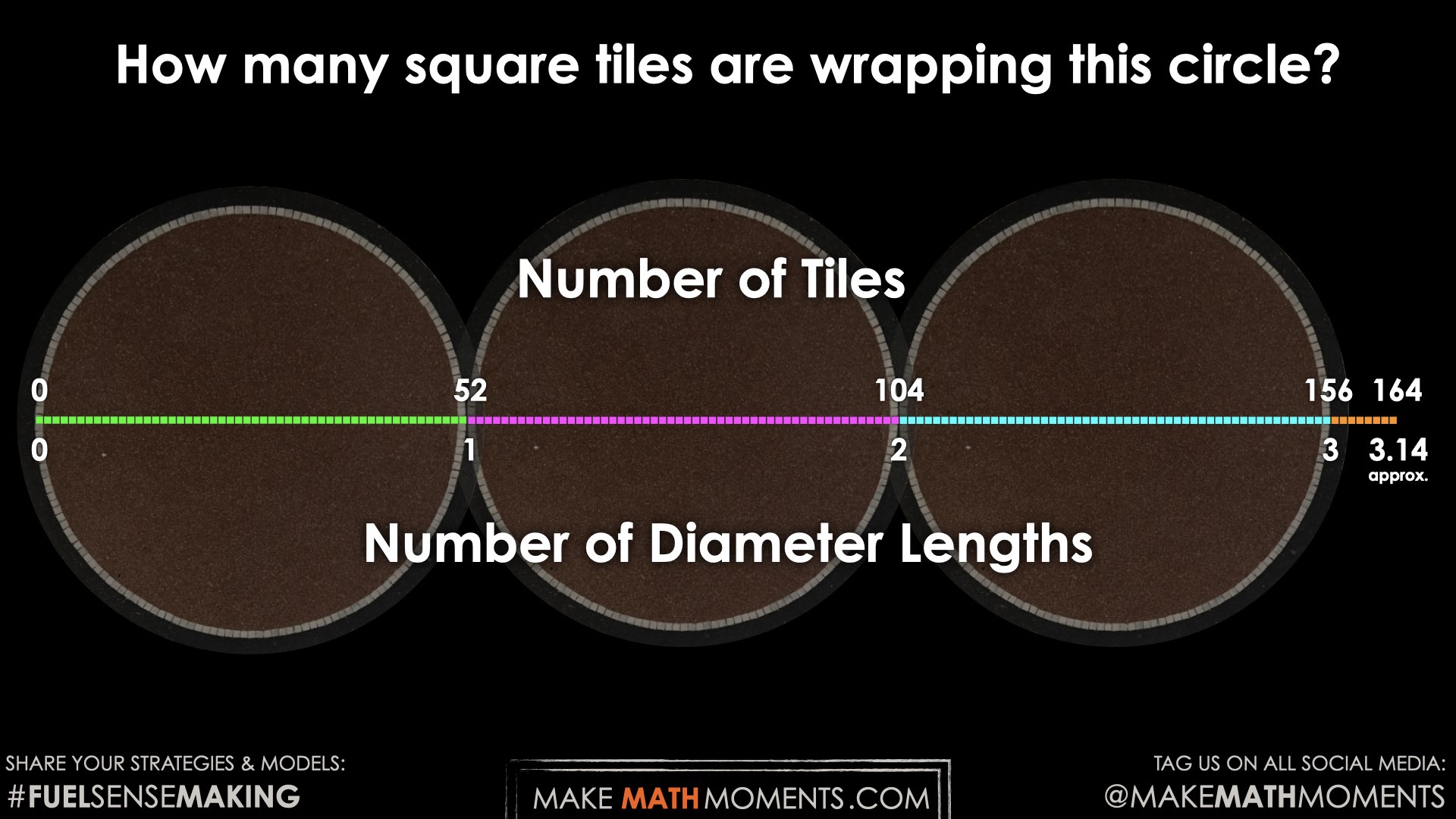
How many square tiles are there around the outer edge of the circle?
Give students an opportunity to make an estimate by first thinking independently and then having them share their estimate with a neighbour.
Ask students to estimate a number of square tiles that they think is too low and a number of square tiles that they think is too high before making their “best estimate”.
After sharing out estimates and recording them on a number line, ask students what information they could use to make their original estimates more precise.
Facilitator Note:
Be sure to reiterate that you want to demote one-to-one counting here. This might mean that you do not leave the image of the entire circle up on the screen, but rather leave the image showing the upper portion of the circle to promote additive (skip counting, repeated addition, etc.) or multiplicative thinking (doubling, tripling, etc.)
Fuel Sense-making
Crafting A Productive Struggle: Prompt
After they brainstorm and share out, show the video below:
Follow up with:
Update your estimate.
How might we convince someone that the quantity you come up with is reasonable without the use of a calculator?
Students have now been given enough information to now make calculations using mental math to improve their estimates based on spatial reasoning and/or the prior knowledge they acquired from the investigation that took place comparing circumference to the diameter from the previous day.
During Moves
While Students Are Productively Struggling…
Login/Join to access the entire Teacher Guide, downloadable slide decks and printable handouts for this lesson and all problem based units.
Student Approaches
Student Approach #1: Concrete Using Connecting Cubes
Login/Join to access the entire Teacher Guide, downloadable slide decks and printable handouts for this lesson and all problem based units.
Student Approach #2: Concrete Using String
Login/Join to access the entire Teacher Guide, downloadable slide decks and printable handouts for this lesson and all problem based units.
Student Approach #3: Using Multiplicative Comparison Between Diameter and Circumference (Pi, π)
Login/Join to access the entire Teacher Guide, downloadable slide decks and printable handouts for this lesson and all problem based units.
Next Moves
Reveal
After students are finished sharing what they noticed from their exploration, share what really happened by showing the following video:
Here is a screenshot from the final frame of the reveal video:
Answer: 164 square tiles.
Consolidation
Login/Join to access the entire Teacher Guide, downloadable slide decks and printable handouts for this lesson and all problem based units.
Reflect and Consolidation Prompts
Provide students an opportunity to reflect on their learning by offering these consolidation prompts to be completed independently.
Consolidation Prompt #1:
Login/Join to access the entire Teacher Guide, downloadable slide decks and printable handouts for this lesson and all problem based units.
Consolidation Prompt #2:
Login/Join to access the entire Teacher Guide, downloadable slide decks and printable handouts for this lesson and all problem based units.
Consolidation Prompt #3:
Login/Join to access the entire Teacher Guide, downloadable slide decks and printable handouts for this lesson and all problem based units.
Consolidation Prompt #4:
Login/Join to access the entire Teacher Guide, downloadable slide decks and printable handouts for this lesson and all problem based units.
We suggest collecting this reflection as an additional opportunity to engage in the formative assessment process to inform next steps for individual students as well as how the whole class will proceed.
Resources & Downloads
Login/Join to access the entire Teacher Guide, downloadable slide decks and printable handouts for this lesson and all problem based units.
Educator Discussion Area
Login/Join to access the entire Teacher Guide, downloadable slide decks and printable handouts for this lesson and all problem based units.
Explore Our 60+ Problem Based Units
This Make Math Moments Lesson was designed to spark curiosity for a multi-day unit of study with built in purposeful practice, number talks and extensions to elicit and emerge strategies and mathematical models.
Dig into our other units of study and view by concept continuum, grade or topic!
