DELICIOUS DONUTS
MAKING FIVE
Kindergarten lesson on decomposing numbers up to five..
Intentionality
Spark Curiosity
Fuel Sensemaking
During Moves
Student Approaches
Next Moves
Consolidation
Reflect and Consolidation Prompts
Resources & Downloads
Educator Discussion Area
Intentionality & Unit Overview
Length of Unit: 5 Days
Access each lesson from this unit using the navigation links below
Students will investigate how many different combinations of donuts there might be on a plate.
Intentionality…
In this task, students will determine the different combinations of donuts that could be in the box. The intention of this task will be to build knowledge and understanding of combinations of 5.
Some of the big ideas that may emerge through this task include:
- There are many different ways to make 5;
- Communication of counting and grouping strategies using manipulatives, words, numbers, and picture;
- Understanding that there are different ways to combine and separate numbers to make 5
Spark Curiosity
What Do You Notice? What Do You Wonder?
Show students this video: Then, ask students:What do you notice?
What do you wonder?
Give students 2-5 minutes to draw on their white board what they noticed and wondered..
Replaying the video can be helpful here.
Then, ask students to share with a peer (Turn and Talk) to share what they noticed (allow 1-2 minutes).
Some of the noticing and wondering that may come up includes:
- I see lots of donuts.
- I see sprinkle donuts.
- I see so many sprinkles.
- I noticed there were different kinds of donuts.
- I wonder who the donuts are for.
- I wonder who made the donuts.
- I noticed that some donuts were chocolate and some had sprinkles.
- I noticed the donuts are the same size.
- The donuts were made in a bakery.
- The donuts are for a birthday party.
Estimation: Prompt
After we have heard students and demonstrated that we value their voice, we can land on the first question we will challenge them with:
How many donuts do you think could fit on the plate?
(use a plate or tray/box that fits 5 donuts)
(place 1 donut on plate for reference)
Make an estimate
We can now ask students to make an estimate (not a guess) as we want them to be as strategic as they can possibly be. This will encourage them to think about the size and number that will fit.
Consider asking students to think about a number of donuts that would be “too low” and a number that would be “too high” before asking for their best estimate in order to help them come up with a more reasonable estimate.
Encourage students to share their estimates, however avoid sharing their justification just yet. We do not want to rob other students of their thinking.
Estimation: Update & Partial Reveal
Show the following video: Ask students to update their estimate.
Facilitator Note:
Educators could provide children with playdough, math manipulatives or loose parts, or a whiteboard to show their thinking.
While students are updating their estimate, listen and observe as students work to make updates. What strategies and mathematical models are students leveraging? Are they:
- Visualizing (e.g., touching to count where they see donuts)
- Drawing an image that represents how the could fit?
- Thinking about proportion? (e.g., drawing tiny or large donuts)
- Using spatial reasoning?
- How are they organizing? (e.g., rows, scattered)
Fuel Sense-making
Crafting A Productive Struggle: Prompt
Now that we know that 5 donuts can fit on the plate/tray, prompt students by stating:
We can fit 5 donuts on the plate. We want to buy a mixture of chocolate and sprinkle donuts. How many of each donut could fit on the plate?
During Moves
While Students Are Productively Struggling…
Problem solving could be presented in a whole or small group learning experience depending on the dynamics and routines in your classroom.
As children are solving the problem, provide them with manipulatives and tools that will support them in visualizing and building different combinations of 5.
Monitor student thinking by observing and documenting what the children are saying and doing. Select and sequence some of the student solution strategies and ask a student from the selected groups to share with the class from:
- most accessible to least accessible solution strategies and representations;
- most common/frequent to least common/frequent strategies and representations; or,
- choose another approach to selecting and sequencing student work.
The tools and representations you might see students using to convince their peers and/or the teacher include:
- loose parts (2 colours)
- flip chips
- 5 frame
- whiteboard and marker
- paper and pencil
- playdough
Have students share their strategies and reasoning for how to represent the different combinations that they found. Ask them to convince you and their peers that their answer is correct by sharing mathematical models.
Discuss their strategies and elicit student thinking during your consolidation to build off of their current prior knowledge and understanding rather than “fixing” or “funnelling” student thinking to a strategy and/or model that does not connect to their strategy and/or approach.
Student Approaches
Student Approach #1: Using Playdough (one solution) – Counting On
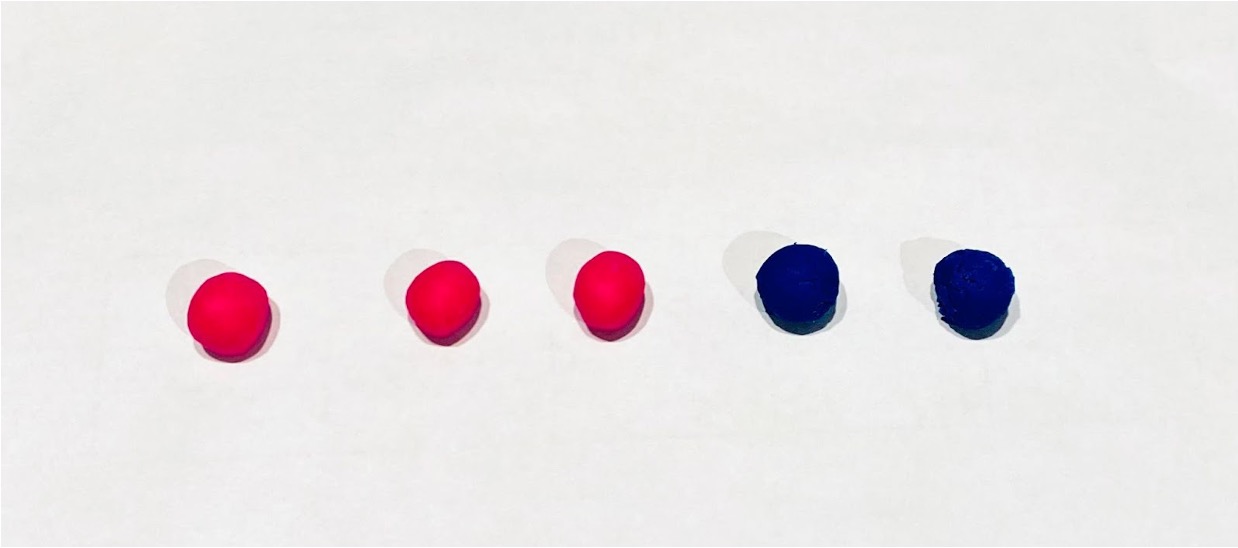
I added 3 chocolate donuts. Then I counted on and added 2 more. There were 5 altogether.
Student Approach #2: Using 5 Frame
I put 2 chocolate in the frame. Then I counted 3 spots that are empty. 2 and 3 makes 5.
Next Moves
Reveal
Show students the following reveal video:
Consolidation
You might consider selecting student solutions that include multiple solutions, instead of just one combination. We want to highlight for students that there is not just one answer to the problem, but many combinations that can be made.
The 5 frame is a tool that can support children in visually seeing the different combinations and making connections to the anchors of 5 and 10 (e.g., if you have 9 sprinkles, how many spaces are empty (chocolate)?)
The 5 frame is a powerful and important tool for children to become familiar with using.
“Because the number 10 plays such a large role in our numeration system and because two fives equal 10, it is very useful to develop relationships between the numbers 1 to 10 and the benchmark numbers of 5 and 10.”
-Van de Walle
Teaching Student-Centered
Mathematics, v1, pg 126
VIDEO: Show combination on 5 frame, then change and record the total.
(e.g., 1 and 4, changes to 2 and 3).
At this point, it is a great idea to ask students the following prompt.
Prompt:
What tools did you use and how did they help you?
Give students the opportunity to turn and talk or share their responses in the whole group. Some students may say that they used counting, addition, subtraction, visualization, or physical representation (e.g., manipulatives).
Reflect and Consolidation Prompts
Provide students an opportunity to reflect on their learning by offering these consolidation prompts to be completed independently.
Consolidation Prompt #1:
What were some of the different ways that we could have solved the problem with the donut box? (brainstorm with small group or whole class to revisit that problems can be solved in different ways)
Consolidation Prompt #2:
Which model and/or strategy shared during today’s consolidation did you find most helpful? Explain.
Consolidation Prompt #3:
Create your own problem like we did with the donut box. What materials or toys could you use from the classroom?
Solve your problem and be sure to clearly indicate your strategy and model to convince others of your thinking.
We suggest collecting this reflection as an additional opportunity to engage in the formative assessment process to inform next steps for individual students as well as how the whole class will proceed.
Resources & Downloads
Printable Lesson Plan PDF
Videos, Images & Media Files
Apple Keynote Presentation
Powerpoint Presentation
Printable Consolidation Prompts
Educator Discussion Area
Login/Join to access the entire Teacher Guide, downloadable slide decks and printable handouts for this lesson and all problem based units.
Explore Our 60+ Problem Based Units
This Make Math Moments Lesson was designed to spark curiosity for a multi-day unit of study with built in purposeful practice, number talks and extensions to elicit and emerge strategies and mathematical models.
Dig into our other units of study and view by concept continuum, grade or topic!
