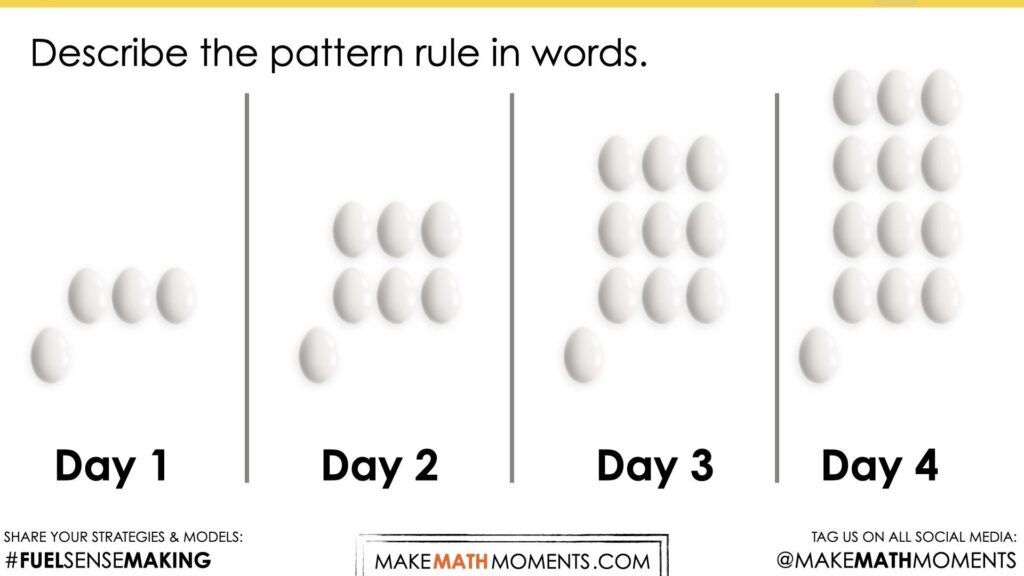
Present the following visual patterns one at a time. For each pattern, ask students to describe the pattern rule in words, including the initial values. In order to determine the initial value, students are encouraged to determine the value of term 0.
Ask students to predict the value of the 8th term and the 14th term. Students are encouraged to justify their thinking. Look for opportunities to build efficiency. For example, once students have determined the rate, and the initial value, rather than counting on or repeated addition, can students leverage this information to perform a calculation?
For example: In the first pattern, the rate is 3, and the initial value is 1. Students can multiply the term number by 3, and add 1. Term 5 multiplied by 3 is 15, plus 1 is 16.
Begin playing the following visual number talk prompt video and be ready to pause the video to allow for think time.
After the visuals for the first two days in the visual pattern appear, pause the video and share the following prompt:
Use manipulatives or draw what you think might come next.
After giving students enough time to create (using square tiles, unifix cubes, etc.) or draw what might come next for Day 3, ask them to share their thinking with their neighbour before sharing out to the class.
Before resuming the video to reveal what the figure for Day 3 looks like, be sure to celebrate the different possibilities shared encouraging them to explain their thinking. It is important to also explicitly explain that while all of the figures shared are all possible, we are going to explore a specific pattern that has a Day 3 figure that looks like this.
If any students in the room had successfully anticipated the figure for Day 3, be sure to celebrate and ask students to recall the thinking that those students had shared previously to help them predict what they think might come next for Day 4.
Student Prompt:
What might come next for Day 4?
Create a figure using manipulatives or by drawing.
Similarly, give students an opportunity to share their thinking with each other. Pay close attention to which students are already noticing a linear growing visual pattern forming and encourage them to continue to verbalize how they see the pattern growing prior to revealing the next figure.
Although you may have already encouraged some students to verbalize how they see the visual pattern growing, be sure to explicitly have all students now:
Describe the pattern rule in words.
It can be helpful to frame this request using a script such as the following:
Pretend that you are on the phone with another student who isn’t present today and cannot see the pattern we are all viewing right now. How would you communicate how they should create each of the first four days in the pattern?
At this point, we hope that students are able to articulate the growing visual pattern as something similar to:
Begin with 1 and increase by 3 each time.
Be prepared for some students to say something like:
Begin with 4 and increase by 3 each time.
However, the true initial value of this linear growing pattern is actually 1 and not 4 since the true “beginning” of this pattern is on Day 0, with 3 less than Day 1.
At this point, we can challenge students to use their visual pattern rule to:
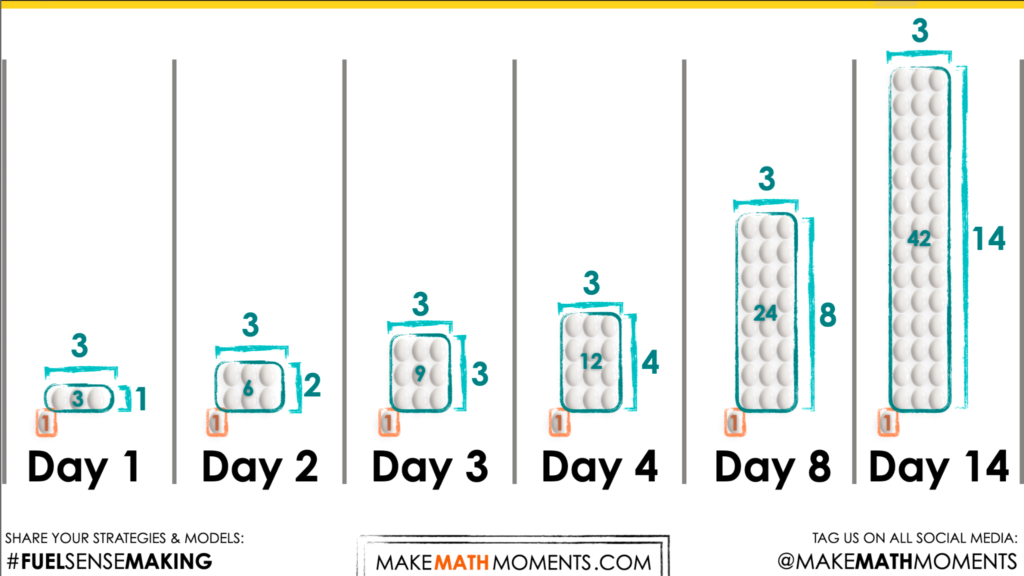
What will Day 8 look like?
What will Day 14 look like?
Describe how you could construct each figure without simply giving the total number of eggs in each figure
At this point, the goal is that students are able to articulate this visual pattern growing at a rate of 3 eggs per day after beginning with a single egg.
Some students might articulate Day 8 as something like:
8 rows of 3 eggs forming a rectangle with a single egg off of the bottom left corner of the rectangle.
Of course, writing out an algebraic expression for this pattern (i.e.: 1 + 3d) or an algebraic equation (i.e.: N = 1 + 3d) can be helpful.
Begin playing the following visual number talk prompt video and be ready to pause the video to allow for think time.
After the visuals for the first two days in the visual pattern appear, pause the video and share the following prompt:
Use manipulatives or draw what you think might come next.
As was shared in the first visual pattern, encourage students to articulate what figure they constructed either with manipulatives or by drawing. Encouraging them to explain without showing their figure can be helpful to promote their adaptive reasoning skills.
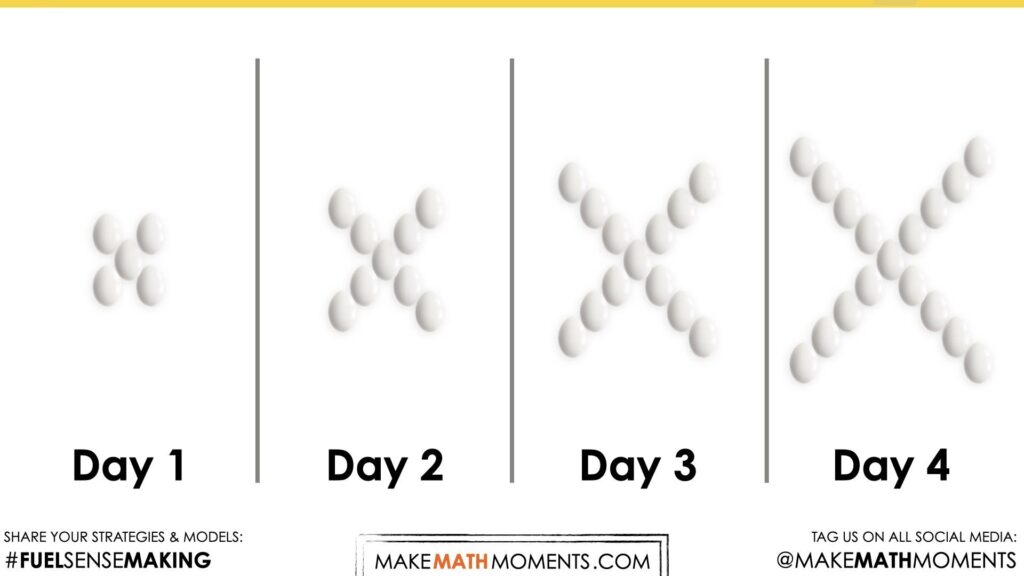
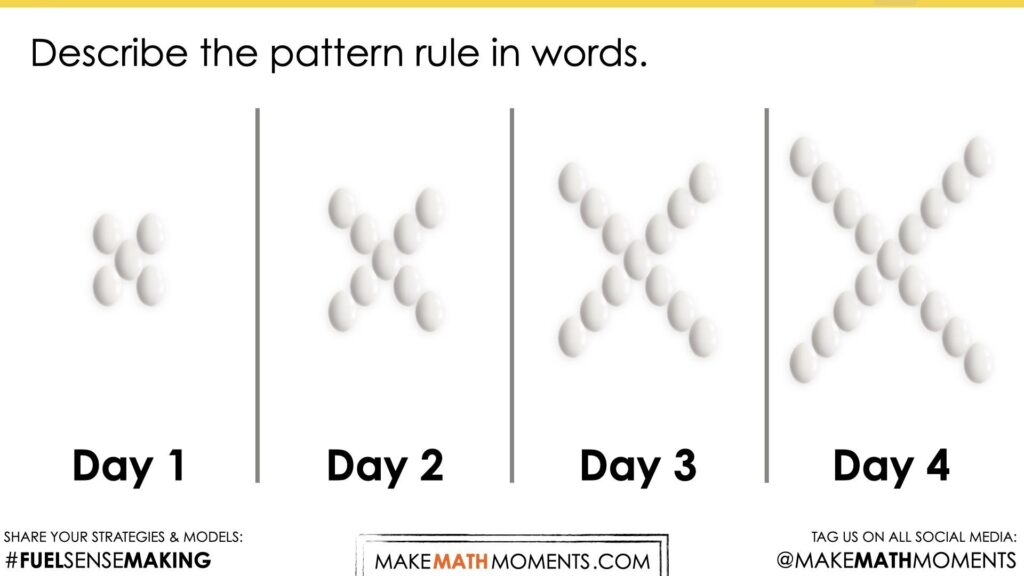
After revealing the figure for Day 3, repeat the process encouraging students to anticipate what might be coming next on Day 4.
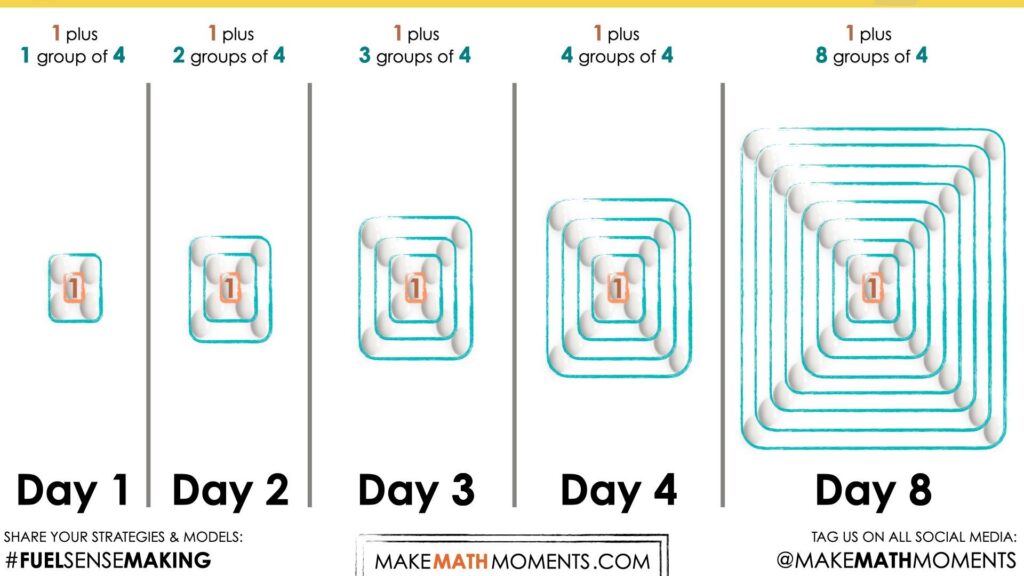
At this point, some students may be able to easily articulate that each of the “ends” are increasing by 1 egg. Other students might be able to highlight the initial value (1 egg) and the rate of change (4 eggs).
Be sure to explicitly state:
Describe the pattern rule in words.
You might once again have them explain it as though they were “painting a picture” verbally over the phone.
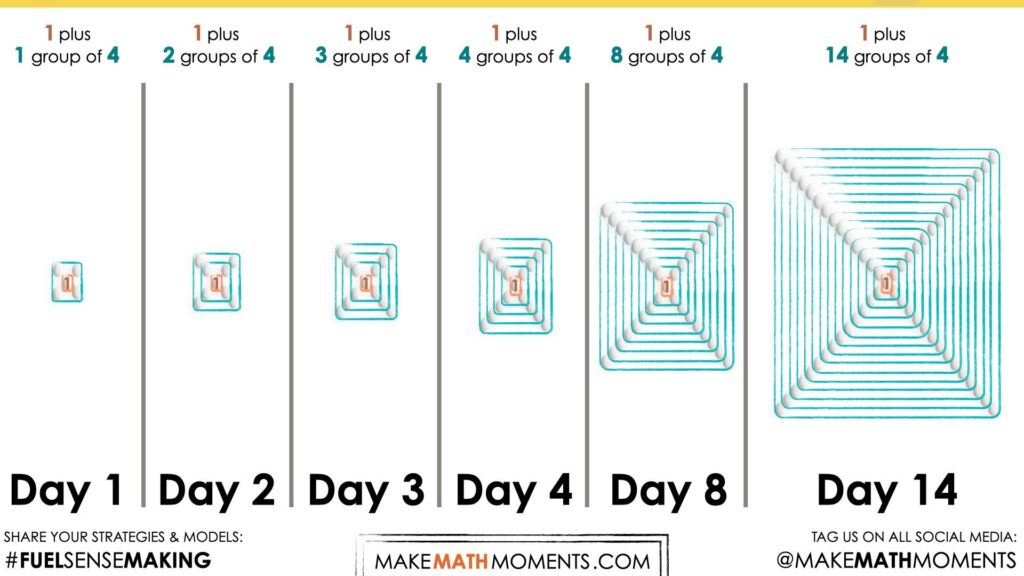
Again, we will have students describe what day 8 and day 14 will “look” like to not only encourage articulating the initial value and rate, but also to use positional language as they must articulate how they see the visual pattern growing.
While many students may be able to visualize this pattern growing, they may have a more difficult time trying to articulate this growth.
Explanations might sound something like:
1 egg plus 4 groups equivalent to the day number.
The 1 egg is in the centre and every day a new egg appears diagonally outward from the centre.
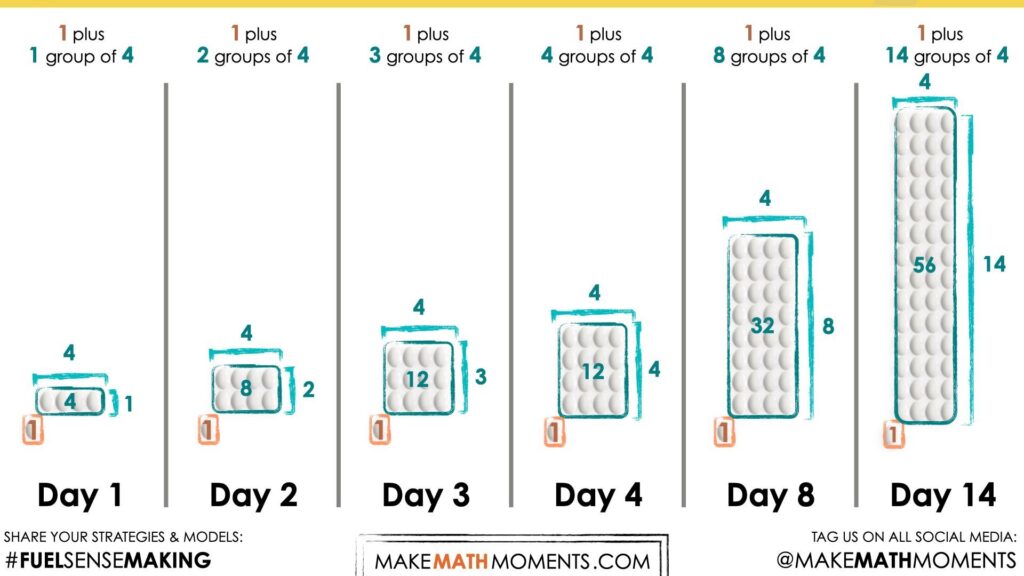
It might be helpful to rearrange the eggs into an array with a leftover egg to make the pattern rule more obvious for all.
Following the pattern rule we created and used above for day 8, students can then determine the number of eggs on day 14 by determining 14 groups of 4 and adding 1.
Of course, some students may not know the multiplication fact 14 x 4, so leveraging the distributive property to create partial products can be helpful.
For example, some students may choose to decompose the 14 groups into 10 groups and 4 groups:
14 x 4 + 1 = (10 + 4)(4) + 1
= (10)(4) + (4)(4) + 1
= 40 + 16 + 1
= 56 + 1
= 57 eggs
Of course, students could decompose into other partial products as well such as decomposing the rate of 4 eggs per group into 2 + 2 or in any other way to make multiplying 14 by 4 more accessible without the use of a calculator.
Once again, writing out an algebraic expression for this pattern (i.e.: 1 + 4d) or an algebraic equation (i.e.: N = 1 + 4d) can be helpful.
Login/Join to access the entire Teacher Guide, downloadable slide decks and printable handouts for this lesson and all problem based units.
Download the videos, images, and related media files to your computer to avoid streaming.
Download in Apple Keynote format to avoid streaming video and run the lesson smoothly.
Download in Microsoft PowerPoint format to avoid streaming video and run the lesson smoothly.
