JUMPING FROGS
PROBABILITY AND PROPORTIONAL REASONING
Use ratio and rate reasoning to predict the results of a probability experiment.
Intentionality
Spark Curiosity
Fuel Sensemaking
During Moves
Student Approaches
Next Moves
Consolidation
Reflect and Consolidation Prompts
Resources & Downloads
Educator Discussion Area
Intentionality & Unit Overview

Current Length of Unit: 1 Day*
*This lesson is the first of an expected 5-day unit. The remaining four (4) days will be released as soon as they are available.
Students will calculate the experimental probability of successfully “flicking” a frog into the container in order to predict how many frogs out of 50 “flicks” we should expect to get in.
The purpose of this Task is:
- to help students develop an understanding of experimental probability; and,
- to apply an understanding of proportional reasoning to scale the ratio comparison of number of successes and total number of attempts using a double number line and a ratio table.
Intentionality…
As is true for any task, the intentionality or learning objective can vary depending on what mathematical thinking you are hoping to elicit. The mathematical ideas we are trying to elicit when we use this task are to bring about the idea of comparing experimental probability with theoretical.
We’ll also use a sample probability to predict the results of the population using proportional reasoning.This will be achieved by first having students seeing how many frogs are successfully flipped into a container out of a set number of trials. Students will use proportionality to extrapolate how many “successes” will occur in 52 trials.
To give yourself a foundation and confidence for discussing proportional reasoning with your students we suggest you complete the course The Concept Holding Your Students Back as we dive into these ideas in depth.
Spark Curiosity
What Do You Notice? What Do You Wonder?
Show students this video: Ask students to engage in a notice and wonder protocol. Anything and everything that comes to mind is fair game. Here’s some of the responses students shared and we would write on chart paper, chalkboard, or whiteboard:- I notice frogs being flipped into a bucket.
- I notice a cat.
- I notice there are different colours.
- I wonder why we’re watching this?
- I wonder how many frogs he makes in the bucket?
- I wonder if I can beat him at this game?
- And many others…
Prompt & Estimate
Now share the prompt that we will use as a jumping off point for this task:How many frogs did he get in the bucket? Make an estimate.We can now ask students to use their estimation skills to come up with a number they feel is too low, a number they feel is too high, and one that they feel is their best guess. Students will also be uncomfortable here because the total number of frogs has not been revealed yet. We encourage you to hold off on revealing this information because it will build anticipation. This anticipation is what helps develop a high level of curiosity and almost naturally encourages students to want to start formulating a plan.
Update Your Estimate
At this point, we want to give students the opportunity to improve their estimates by engaging in developing a problem solving strategy. Ask students:If we are going to improve our estimates, what information will we need? Take a minute to think independently and then discuss your thoughts with an elbow partner.After students have been given some time to think independently, instruct the students to turn and talk with elbow partners. As the conversation gains momentum in the room, ask students to begin sharing out to the class. While it might feel like you are cutting discussions short, waiting until the discussions end will mean less students will be eager to share with the whole group. As students voice the information they wish to see, ask them:
What would you do with that information if we were able to share that with you?Listen closely here as their responses give you important formative assessment details about their prior knowledge and their thinking into how they might begin solving this problem. After students have shared their “information wishlist” and what they would use the information for, you can reveal this image of the total number of frogs or total number of shots taken:
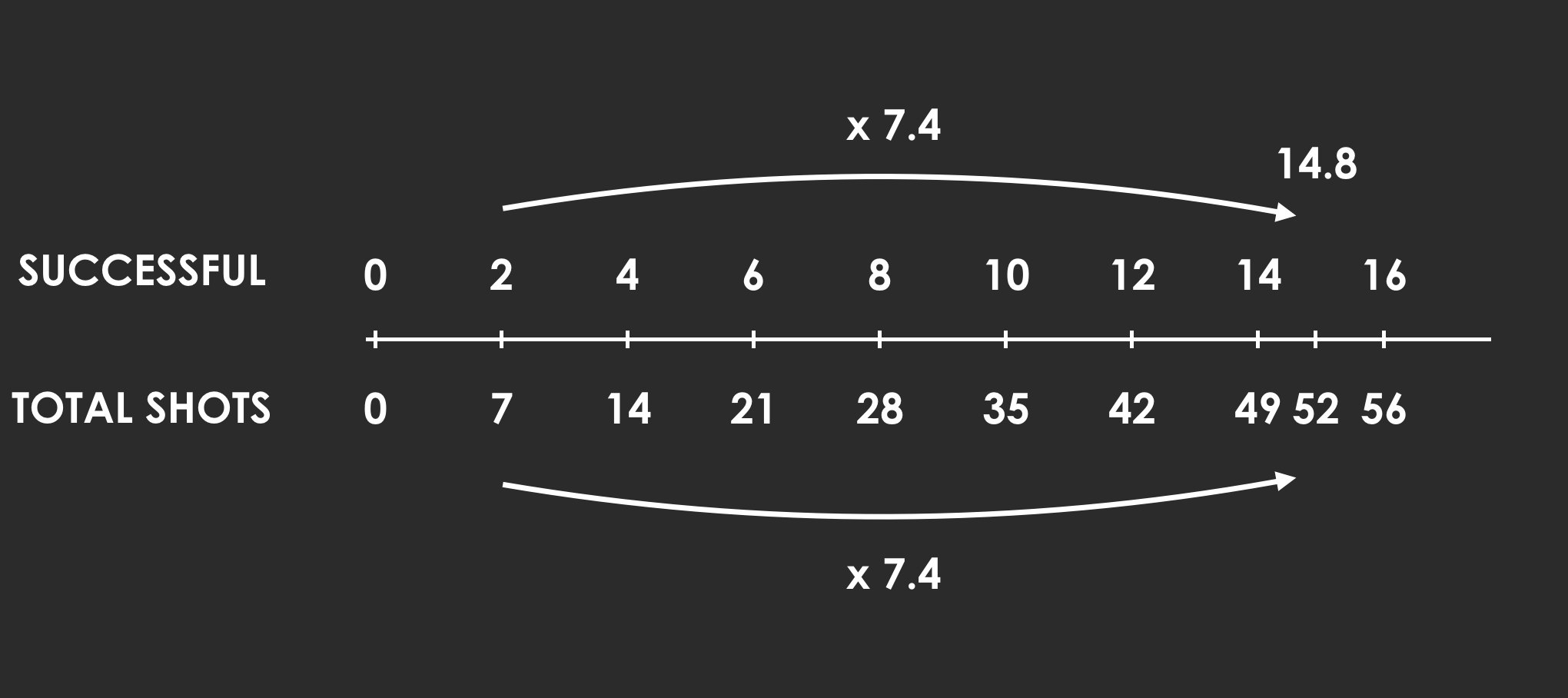
 Revisit the Spark video to clearly point out that in 7 shots this person took, he was successful 2 times.
Facilitator Move:
Consider taking some time to discuss independent events vs. dependent events:
Revisit the Spark video to clearly point out that in 7 shots this person took, he was successful 2 times.
Facilitator Move:
Consider taking some time to discuss independent events vs. dependent events:
Does scoring one shot impact the outcome of the next or is it safe to assume that each trial is independent?Have students share their final updated estimates with their partners and give students an opportunity to share their strategies and conclusions based on the activity and this new information.
Fuel Sense-making
Crafting a Productive Struggle: Prompt
With students aware that the shooter got 2 frogs in the bucket out of 7 shot total, you can ask them to update their estimates by prompting:
How many successes will be made in 52 trials?
Be convincing
Walk Through Video: Anticipating Student Approaches
Watch the following video to help you anticipate what student approaches you might see including strategies and mathematical models.
During Moves
While Students Are Productively Struggling….
Monitor student thinking by circulating around the room and listening to the mathematical discourse. Select and sequence some of the student solution strategies and ask a student from the selected groups to share with the class from:
- most accessible to least accessible solution strategies and representations;
- most common/frequent to least common/frequent strategies and representations; or,
- choose another approach to selecting and sequencing student work.
The tools and representations you might see students using to convince their peers and/or the teacher include:
- Concrete representations (i.e.: ratio of green “success” square tiles to red “fail” square tiles, etc.)
- Double number line.
- Ratio table.
- Symbolic.
Have students share their strategies and reasoning for determining the total number of successes that you would expect given the ratio of successes to total shots of 2 to 7.
Ask them to convince you and their peers that their answers are correct by sharing mathematical models.
Discuss their strategies and elicit student thinking during your consolidation to build off of their current prior knowledge and understanding rather than “fixing” or “funnelling” student thinking to a strategy and/or model that does not connect to their strategy and/or approach.
Student Approaches
Student Approach #1: Double Number Line
I used a number line and a scale factor of about 7 and a half to determine the number of made shots.
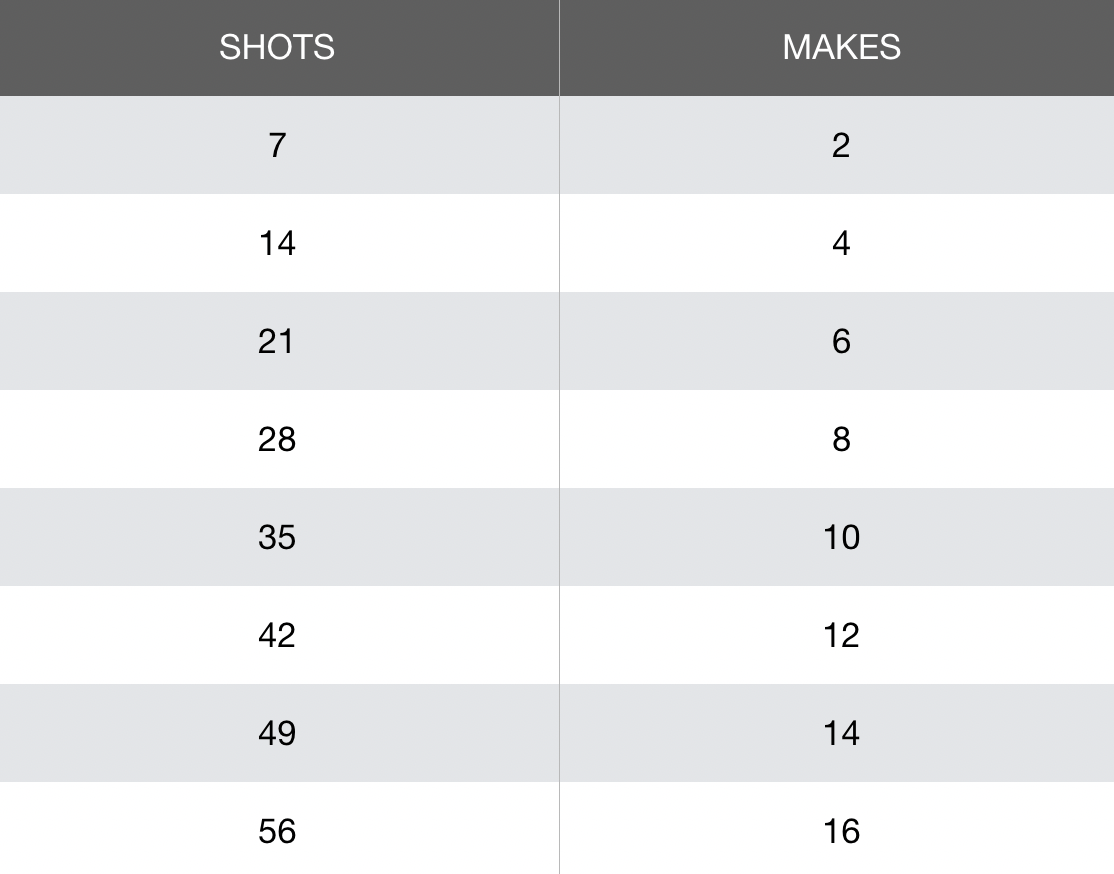
Student Approach #2: Ratio Table
“I created a table to show how the number of made shots relates to the total shots by skip counting by 7 and skip counting by 2.”
Student Approach #3: Symbolic
“I was thinking that the number of 7 shot trials in 52 is about 7.5 since 52 shots divided by 7 shots is equivalent to 7.42 (quotative division).
If he makes 2 out of every 7 shots then he should make 15 shots since 52 total shots is about 7.5 times greater than 7 shots and 15 is 7.5 times greater than 2.”
Student Approach #4:
“I determined the probability 2/7 is about 29%.
I then determined 29% of 52 which is approximately equal to 15.
So in 52 shots he should make 0.29 x 52 or about 15 shots.”
Next Moves
Reveal
After consolidating learning using student generated solution strategies and by extending their thinking intentionally, we can share what really happened with this video:
Consolidation
Have students share their answers from most accessible (possibly responses #1 and #2) to least accessible (possibly responses #3 and #4). The goal of the teacher will be to show the connections among student approaches. Keep in mind that student solutions may reveal both ratio and rate reasoning.
Students used ratio reasoning if they scaled the composed unit of 2 successes and 7 shots up to approximately 15 successes and 52 shots by using quotative division to divide 52 shots by 7 shots for a scale factor of approximately 7.5. Students used rate reasoning if they partitively divide 2 successes by 7 shots (or alternately multiplying 2 successes by one seventh shots) will help students determine the number of successes per shot (i.e.: the rate). Without the use of a calculator, students may find dividing 2 successes by 7 shots difficult and should be prompted to represent this division concretely or pictorially. Students may decide to use a guess and check approach to avoid dividing altogether.
Through this thinking, the two quantities within this ratio always remain distinct (we scale the successful shots and scale the total shots) even though they are being treated as a composed unit. Once the class has had these conversations show them the reveal video.
Reflect and Consolidation Prompts
Provide students an opportunity to reflect on their learning by offering these consolidation prompts to be completed independently.
Consolidation Prompt #1:
Assuming his shot percentage stays contant, pick a total number of shots that would make finding his number of successes easy without the use of a calculator.
Be convincing.
Consolidation Prompt #2:
Assuming his shot percentage stays contant, pick a total number of shots that would require clever strategizing to find the number of successes without the use of a calculator.
Be convincing.
Consolidation Prompt #3:
If the person in the video made 32 successes, how many shots must have been taken?
How do you know?
We suggest collecting this reflection as an additional opportunity to engage in the formative assessment process to inform next steps for individual students as well as how the whole class will proceed.
Resources & Downloads
Printable Lesson Plan PDF
Videos, Images & Media Files
Apple Keynote Presentation
Powerpoint Presentation
Printable Consolidation Prompts
Educator Discussion Area
Login/Join to access the entire Teacher Guide, downloadable slide decks and printable handouts for this lesson and all problem based units.
Explore Our 60+ Problem Based Units
This Make Math Moments Lesson was designed to spark curiosity for a multi-day unit of study with built in purposeful practice, number talks and extensions to elicit and emerge strategies and mathematical models.
Dig into our other units of study and view by concept continuum, grade or topic!