THE WOOLY WORM RACE - PRIMARY
REPRESENTING, COMPARING, ORDERING & ADDING FRACTIONS
Represent, compare, order, and begin adding fractions with like denominators.
Intentionality
Spark Curiosity
Fuel Sensemaking
During Moves
Student Approaches
Next Moves
Consolidation
Reflect and Consolidation Prompts
Resources & Downloads
Educator Discussion Area
Intentionality & Unit Overview

Length of Unit: 5 Days
Access each lesson from this unit using the navigation links below
To crown a champion for the Wooly Worm Race, students will represent and order the distances travelled by each caterpillar.
Intentionality…
In this task, students will represent the distances travelled by each caterpillar using a concrete or visual model to order them from greatest to least. We have intentionally selected a linear context because we are hoping to emerge the bar model and eventually the number line as effective and efficient representations that can be used when working with fractions.
Some of the big ideas that may emerge through this task include:
- Fractions can be represented in a variety of ways;
- How you partition the whole determines the fractional unit (i.e.: partitioning a whole into 6 parts will result in 6 sixth parts);
- As you partition a whole into more parts, the smaller the size of each part;
- As you partition a whole into more parts, the larger the unit fraction (called the denominator in later grades);
- In order to compare two or more fractional quantities, the whole must be the same;
- The count (called the numerator in later grades) indicates the number of parts relative to the number of parts in the whole indicated by the unit fraction (the denominator); and,
- Fractional amounts exist between whole numbers.
Spark Curiosity
What Do You Notice? What Do You Wonder?
Show students the first minute of this video:
Facilitator Note:
In the actual “Wooly Worm Race”, the first caterpillar to the finish line wins. However, in this particular unit, we will be modifying the rules to better suit our learning goal. Be sure to stop the video after the first minute to avoid introducing information that will conflict with the context of this unit.
Then, ask students:
What do you notice?
What do you wonder?
Give students 60 seconds (or more) to do a rapid write on a piece of paper.
Then, ask students to share with their neighbours for another 60 seconds.
Finally, allow students to share with the entire group. Be sure to write down these noticings and wonderings on the blackboard/whiteboard, chart paper, or some other means to ensure students know that their voice is acknowledged and appreciated.
Some of the noticing and wondering that may come up includes:
- I notice caterpillars.
- They are fuzzy.
- There are strings going up the wall.
- One of the caterpillar’s name is Silly Willy.
- I saw one named Scratchy.
- I think this is a competition.
- I saw someone who looked liked like a referee.
- There were at least 18 caterpillars.
At this point, you can answer any wonders that you can cross off the list right away. For example, share that this is in fact a competition. The competition is called “The Wooly Worm Race” and it is held annually in Banner Elk, NC. The winner is awarded to the caterpillar who climbs to the highest point on their string within the allotted time.
Then, share the following video and explain to students that this is a video showing the distance the winning caterpillar climbed:
A screenshot of the final frame of the video is shown below.
Estimation: Prompt
After we have heard students and demonstrated that we value their voice, we can land on the first question we will challenge them with:
How far do you think the winning caterpillar climbed?
We can now ask students to make an estimate (not a guess) as we want them to be as strategic as they can possibly be. This will force them to use spatial reasoning alone to try and come up with an initial estimate and to share it with their neighbours by trying to articulate why they believe their prediction is reasonable.
Note that we have intentionally left out the length of the race track in order to encourage students to use fractional thinking rather than measuring in standard units. It is clear that the winning caterpillar travelled less than the total distance of the track when the race time “ran out”.
Consider asking students to think about a distance that would be “too low” and a distance that would be “too high” before asking for their best estimate in order to help them come up with a more reasonable estimate.
While any and all estimates should be acknowledged and welcomed, it might be helpful to encourage fractional language. For example, if a student gives a decimal quantity for an estimate stating something like “point 8 of the way”, encourage them to articulate what “point 8” means (i.e.: 8 tenths). For young students, they may already have some understanding of the magnitude of decimal numbers based on their exposure to decimals in the world around them. Plan for how you intend to meet students where they are to help them develop a better conceptual understanding of where decimal numbers come from and what they look like concretely or visually (partitioning a whole into tenths, for example).
Let them chat with their neighbours and challenge them to an estimation duel or a math fight.
While Students Are Estimating…
Monitor student thinking by circulating around the room and listening to the mathematical discourse.
Encourage students to use precise mathematical language (including both standard and not standard units of measure) and positional language (in front, behind, on top…) to articulate their defense.
If students’ estimates are unreasonable, encourage them to select a manipulative similar to what they believe the length of the string to be (perhaps linking cubes or a meter stick).
While we hope to engage in learning around fractions including partitioning a whole to represent fractional amounts for the purpose of comparing, we will welcome students who share their estimates in decimal form and will not impose the use of fractions on them. Because the calculator is introduced to students so early in their mathematical journey, many students default to expressing fractional amounts as decimals without necessarily having a deep conceptual understanding of how they work. Later in this lesson and in this unit, we hope to continue building the conceptual underpinnings necessary for becoming flexible with fractions and we encourage you to attempt making connections to decimals and percentages if students bring them up when estimating or sharing their thinking. If students do estimate using decimals, be sure to help students by using appropriate language utilizing place value and the fractional language associated.
For example, when a student intends to share 0.8, they may verbally describe this quantity as “point-eight of the whole”, we can use this opportunity to improve the conceptual understandings of all students by accepting that response, but reiterating as “eight-tenths of the whole”.
Consider reiterating using more conceptually rich language such as:
- 0.7 shared verbally as “zero-point-eight” reiterated as “seven-tenths of the whole”
- 0.75 shared as “zero-point-seven-five” or “point-seventy-five” reiterated as “seventy-five-hundredths of the whole”
- 0.85 shared as “point-eight-five” reiterated as “eighty-five-hundredths of the whole”
Being intentional about the language we use when verbally describing fractional quantities in decimal notation can go a long way to helping make converting between fractions and decimals as well as place value so much more intuitive when students encounter them later in their mathematical journey.
Encourage students to share.
After students have had an opportunity to share their best guess, tell them that you will now be sharing the distances travelled by the top 3 finishers from the championship race.
Fuel Sense-making
Crafting A Productive Struggle: Prompt
Since you have already taken some time to set the context for this problem and student curiosity is already sparked, we have them in a perfect spot to help push their thinking further and fuel sense making.
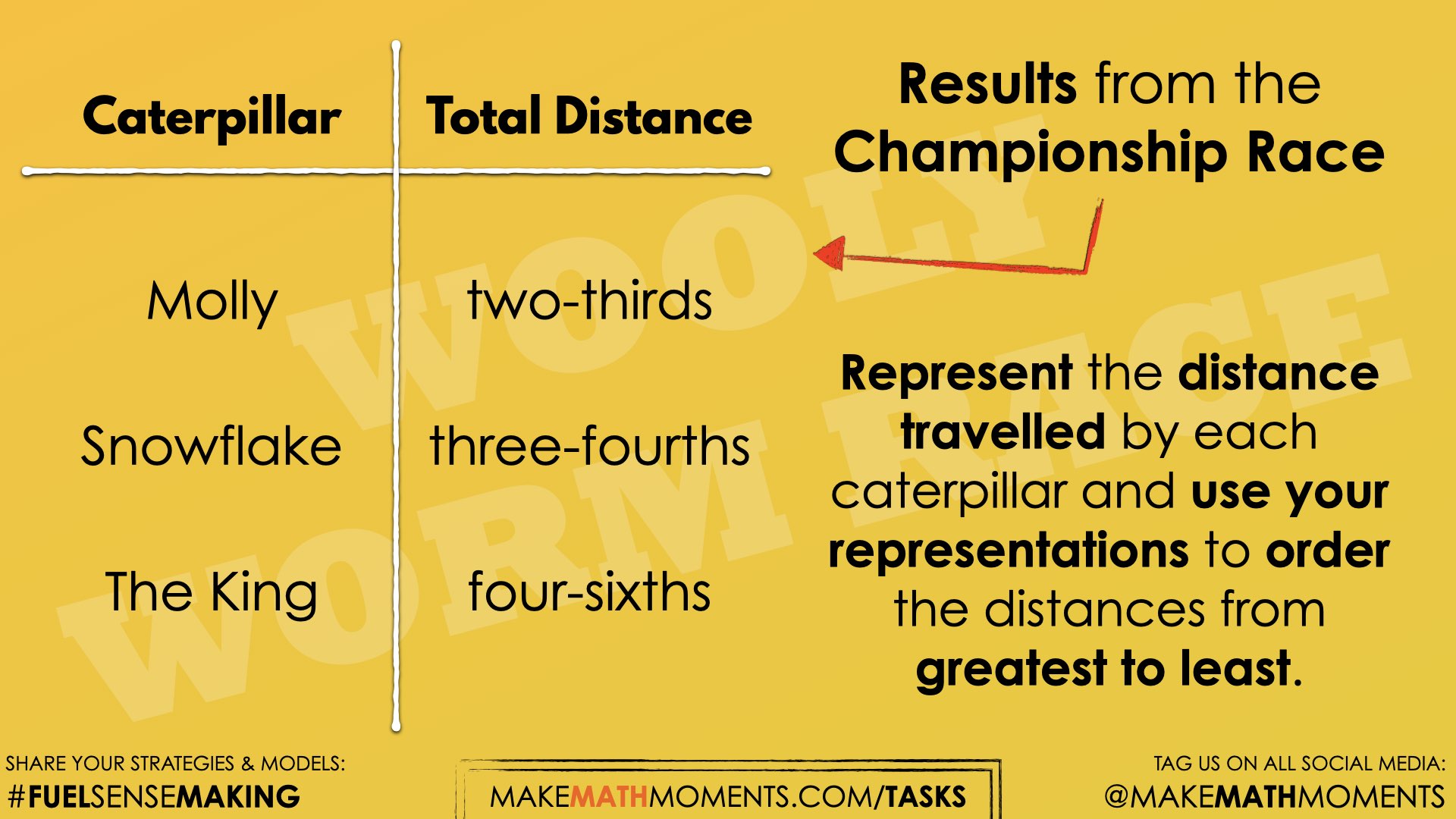
Share the total distance travelled by the top 3 finishers from the championship race:
Notice that the unit fraction is expressed in words rather than standard-notation. We will work towards standard-notation throughout this unit, but for the time being, we want to emphasize the fractional unit. You will also notice that we are only working with a limited number of denominators, and they have been strategically selected.
Prompt students to:
Represent the distance travelled by each caterpillar and use your representations to order the distances from greatest to least.
During Moves
While Students Are Productively Struggling…
Monitor student thinking by circulating around the room and listening to the mathematical discourse. Select and sequence some of the student solution strategies and ask a student from the selected groups to share with the class from:
- most accessible to least accessible solution strategies and representations;
- most common/frequent to least common/frequent strategies and representations; or,
- choose another approach to selecting and sequencing student work.
The tools and representations you might see students using to convince their peers and/or the teacher include:
- Paper folding
- Concrete manipulatives such as fraction strips or relational rods
- A pictorial representation such as a bar model
- A number line (possible, but very unlikely if in a primary classroom)
Have students share their strategies and reasoning for how to represent the total distance travelled by each Wooly Worm. Ask them to convince you and their peers that their answer is correct by sharing mathematical models.
Discuss their strategies and elicit student thinking during your consolidation to build off of their current prior knowledge and understanding rather than “fixing” or “funnelling” student thinking to a strategy and/or model that does not connect to their strategy and/or approach.
Student Approaches
Student Approach #1: Paper Folding
Before starting to solve the problem, we had a discussion as a class to share some possible strategies. My teacher was holding a piece of paper to represent the whole race track, so I decided to cut strips of paper to help me.
I started with Snowflake because I knew it would be easy to fold the paper into 4 equal parts and colour in 3.
For Molly, I tried a couple of times, but the parts weren’t equal. Finally, I figured it out and made 3 equal parts and shaded in 2.
The King was hard. Eventually, I folded a strip of paper into 3 equal parts like I did for Molly and then I folded that in half and it got me 6 equal parts and shaded in 4.
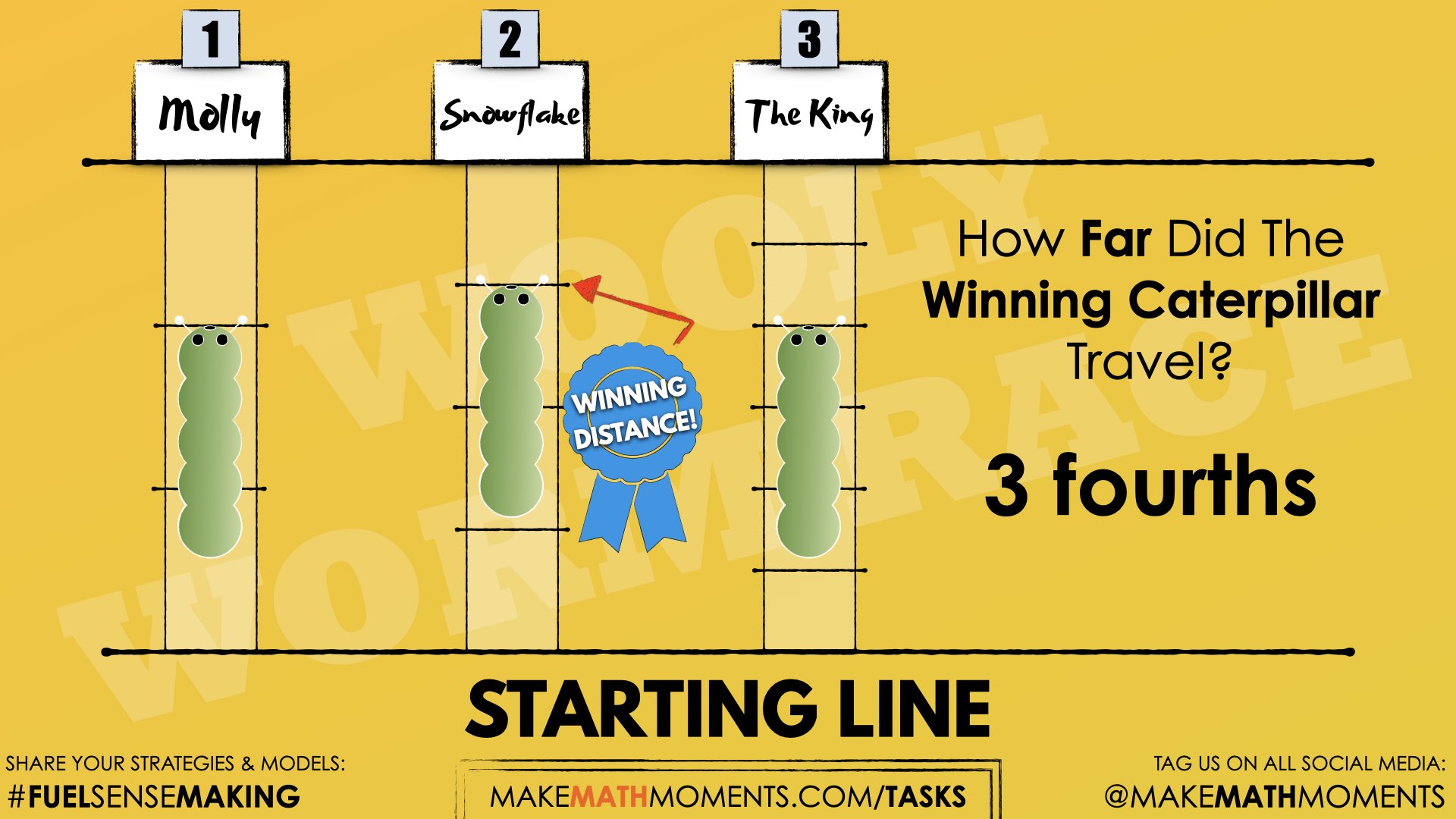
Snowflake definitely won the race and Molly and the King tied for 2nd.
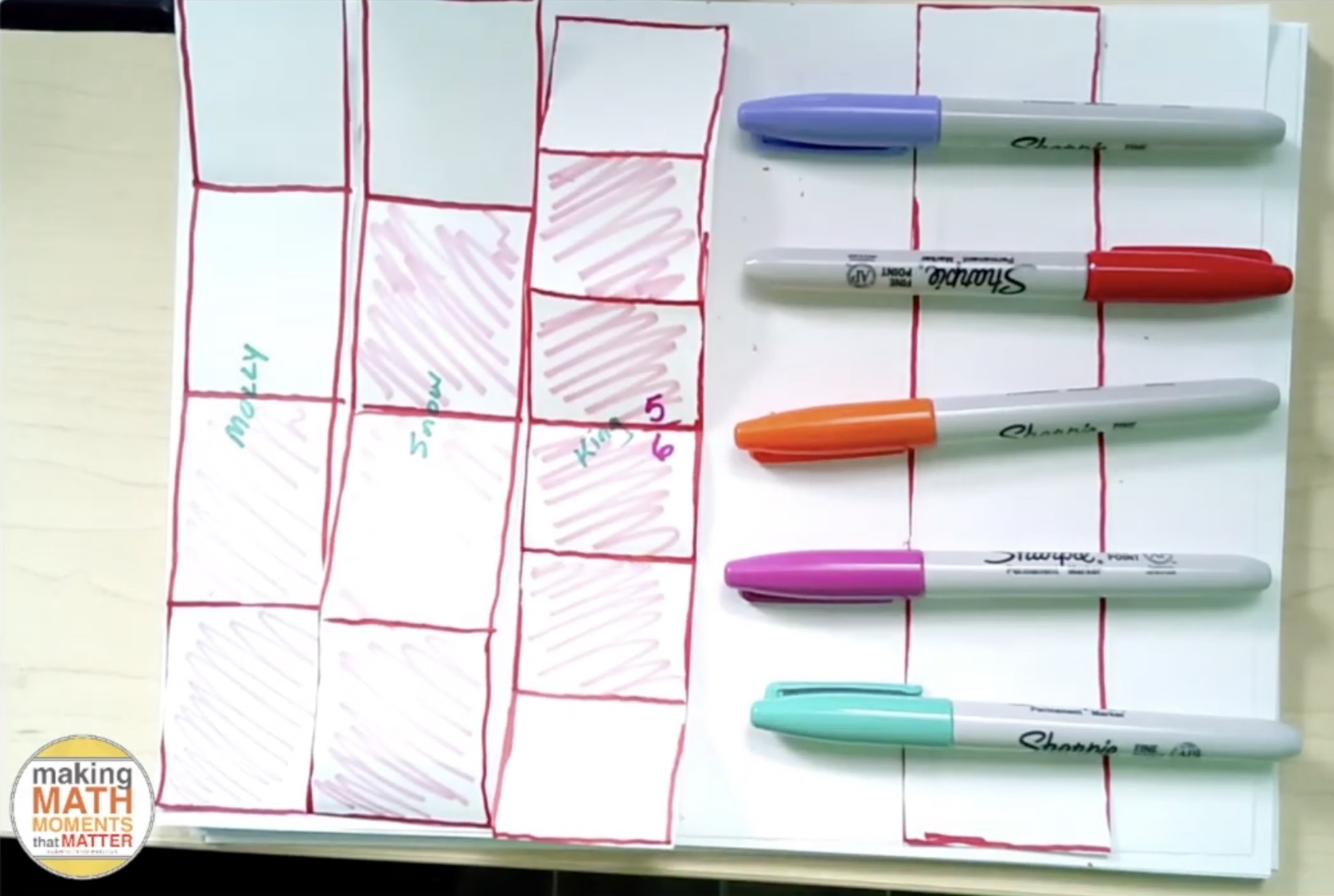
Student Approach #2: Drawing a Bar Model
I drew 3 of the same length rectangles to represent the distance of the race track for the top 3 racers.
I used my markers to help me figure out where to draw the lines to make equal parts for each of the racers.
After colouring in the number of parts for each racer, I noticed that Snowflake went the furthest and Molly and The King tied for 2nd place.
Next Moves
Reveal
fter consolidating learning by making connections using student generated solutions, you can share the following reveal video:
A still frame of the winning caterpillar is as follows:
Go back to student estimates from the Spark portion of the lesson to determine who had the closest estimate. Be sure to celebrate the student or students who were closest so that students realize that there was value in sharing with the group.
Did students use decimal notation and/or decimal language and/or percentages to share estimates at the beginning of the lesson? Consider extending from here to give students an opportunity to explore and make connections with comparing and converting between benchmark fractions, decimals and percentages.
Consolidation
During today’s consolidation, the goal is to reveal the power of the concrete and pictorial linear model as a tool to represent, order, and compare fractions. Rather than leading students towards the idea of finding a common denominator (which could be beyond their level of conceptual understanding at this point), these models are an effective tool to reason through this scenario and demonstrate the skill of comparing and ordering fractions with unlike denominators. Through the consolidation, there is also a potential to emerge the idea of equivalence using different fractional units. For example, Molly and The King reached the same point, but their distance was expressed using a different fractional unit.
The consolidation is your opportunity to address any misconceptions that may exist such as students who may have struggled to equi-partition their wholes, or to be mindful of the consistently congruent distance of the whole race track. Students may need more time to develop this spatial awareness in order to use the pictorial models effectively. Students may have believed that a fraction expressed with a different fractional unit cannot be equivalent. This lesson serves to reveal this big idea.
Reflect and Consolidation Prompts
Provide students an opportunity to reflect on their learning by offering these consolidation prompts to be completed independently.
Consolidation Prompt:
In last year’s competition, Silly Willy travelled a distance of 2 thirds of the whole race track while Yo Gaba Gaba travelled a distance of 3 fifths of the whole race track.
Compare the results of these two caterpillars.
Who travelled the furthest and how can you convince your math community that you’re right?
We suggest collecting this reflection as an additional opportunity to engage in the formative assessment process to inform next steps for individual students as well as how the whole class will proceed.
Resources & Downloads
Printable Lesson Plan PDF
Videos, Images & Media Files
Apple Keynote Presentation
Powerpoint Presentation
Printable Consolidation Prompts
Educator Discussion Area
Login/Join to access the entire Teacher Guide, downloadable slide decks and printable handouts for this lesson and all problem based units.
Explore Our 60+ Problem Based Units
This Make Math Moments Lesson was designed to spark curiosity for a multi-day unit of study with built in purposeful practice, number talks and extensions to elicit and emerge strategies and mathematical models.
Dig into our other units of study and view by concept continuum, grade or topic!