SCAVENGER HUNT
REPRESENT CATEGORICAL DATA AND EXPLORE MEAN
Explore thre graphical representation of categorical data and the use of mean as a measure of central tendency.
Intentionality
Spark Curiosity
Fuel Sensemaking
During Moves
Student Approaches
Next Moves
Consolidation
Reflect and Consolidation Prompts
Resources & Downloads
Educator Discussion Area
Intentionality & Unit Overview
Length of Unit: 4 Days
Access each lesson from this unit using the navigation links below
Students will estimate the amount of candy collected by one participant by observing the emptying of her bag in a video. Students will be asked to represent the quantity of candy collected by the other five participants graphically relative to the one featured in the video clip.
Intentionality…
In this task, students will explore estimation, relative thinking and the graphical representation of categorical data. Some of the big ideas that will be explored in today’s task include:
- One quantity can be compared relative to another.
- Value bars and other graphical representations can be used to show relations.
- There are different categories of data (i.e. categorical, numerical, continuous..). The category should help determine how the data is represented
Spark Curiosity
What Do You Notice? What Do You Wonder?
Show students this video: You may want to pause the video or keep the following image up on the screen:
In this context, a girl is dumping out candy from a bag.
Then, ask students:
What do you notice? What do you wonder?Give students 60 seconds (or more) to do a rapid write on a piece of paper. Then, ask students to share with their neighbours for another 60 seconds. Finally, allow students to share with the entire group. Some of the noticing and wondering that came up in a class recently included:
- There is a girl with candy.
- She is dumping candy on the counter.
- She is in the picture with a boy in the background.
- Clean kitchen.
- I wonder what kind of candy that is?
- I wonder how many candies there are?
- Why is she dumping all of the candy on the counter?
- What is her name?
- How old is she?
- As well as others…
Estimation: Prompt
After we have heard students and demonstrated that we value their voice, we can land on the first question we will challenge them with:Follow up that question with:How many candies were in the bag?
We can now ask students to make an estimate (not a guess) as we want them to be as strategic as they can possibly be. This will force them to use spatial reasoning alone to try and come up with an initial estimate and to share it with their neighbours by trying to articulate why they believe their prediction is reasonable.How might we convince someone that the quantity you come up with is correct?
Consider asking students to think about a number that would be “too low” and a number that would be “too high” before asking for their best estimate in order to help them come up with a more reasonable estimate.
Let them chat with their neighbours and challenge them to an estimation duel or a math fight.
While Students Are Estimating…
Monitor student thinking by circulating around the room and listening to the mathematical discourse. Encourage students to use precise mathematical language (including greater than, less than, more, less, half, rows…) and positional language (in front, behind, on top…) to articulate their defense. If students’ estimates are unreasonable, encourage them to select a manipulative similar in size of the candy (perhaps a linking cube) and ask them to lay out that number of items in front of them to consider whether that quantity seems reasonable. Allowing students to share their estimates with neighbours first, then with the class. Write down their estimates on the chalkboard/whiteboard/chart paper so students feel their voices are being heard and so they feel they have a stake in solving this problem. Tell students that you will not be sharing the exact quantity just yet, however, the number that they estimated will be useful.Fuel Sense-making
Walk Through Video
Consider watching this video as a guide through the Sense Making portion of this task.Share The Context: The Scavenger Hunt
Explain the context behind the video by sharing this story:Lucia invited five friends over to celebrate her birthday. She planned a variety of activities for the day, one of them being a scavenger hunt. Lucia and her friends headed to her neighbourhood park to complete the challenge. Equipped with a list of clues, all six friends headed off in different directions to follow the clues to each checkpoint. There were 12 different clues and corresponding locations along the scavenger hunt. Every time a participant found a location, there was a bucket full of candy. Participants could take a handful of candy from all 12 stops. At the end of the scavenger hunt, they returned to Lucia’s and emptied their bags of candy onto the floor. The friends quickly realized that they did not have an equal distribution of candy in their bags.
Crafting A Productive Struggle: Prompt
Since you have already taken some time to set the context for this problem and student curiosity is already sparked, we have them in a perfect spot to help push their thinking further and fuel sense making. Share the following information about the candy collected by each participant:After they dumped their candy on the floor, the friends compared how much candy they collected with the rest of the group. They found that:
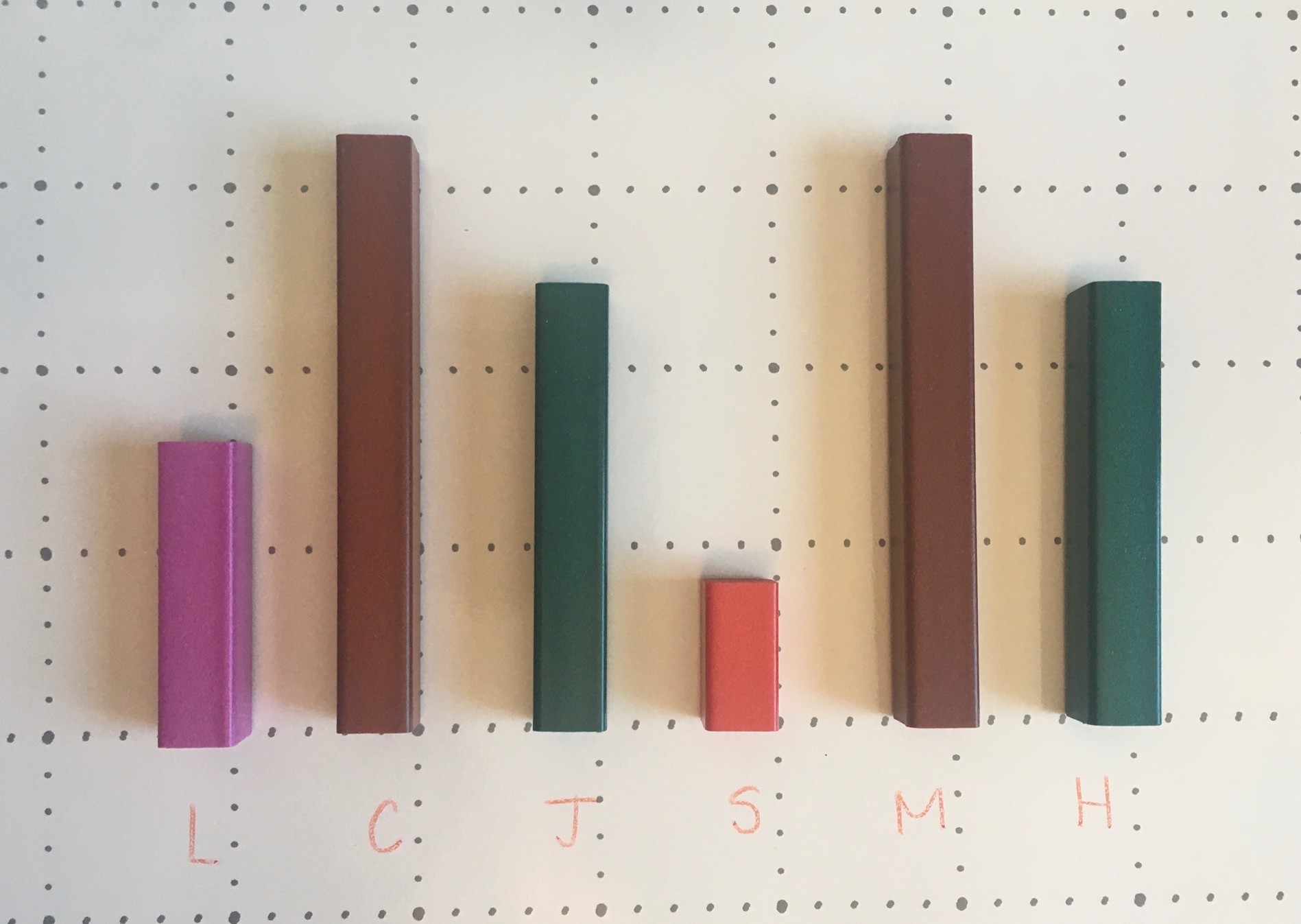
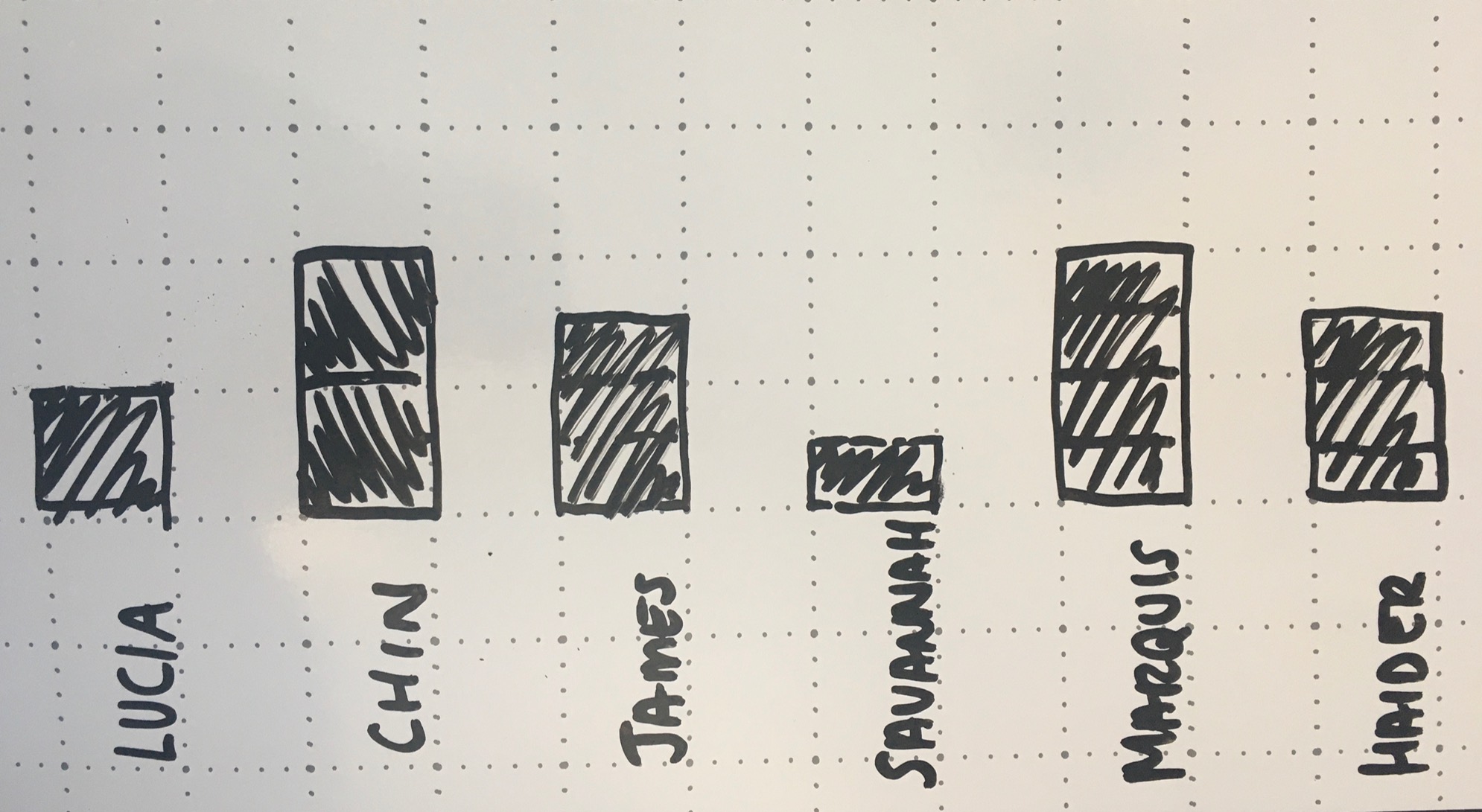
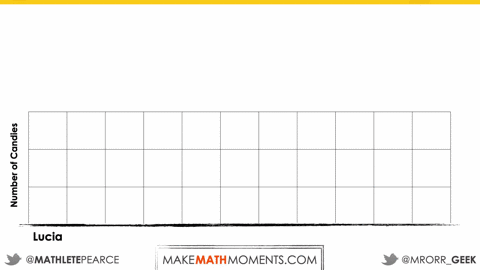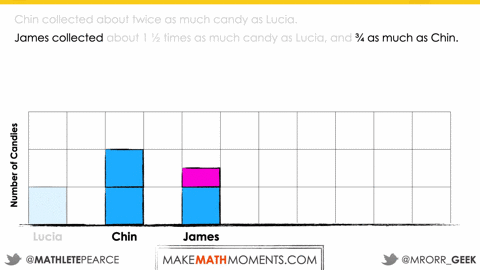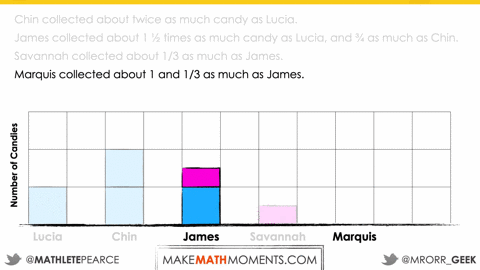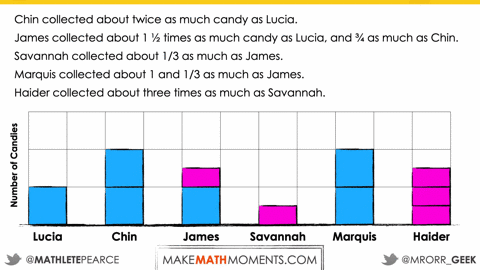
- Chin collected about twice as much candy as Lucia.
- James collected about 1 ½ times as much candy as Lucia, and ¾ as much as Chin.
- Savannah collected about 1/3 as much as James.
- Marquis collected about 1 and 1/3 as much as James.
- Haider collected about three times as much as Savannah.
Create a graphical or visual representation to compare the quantity of candy collected by each participant.
During Moves
While Students Are Productively Struggling….
Monitor student thinking by circulating around the room and listening to the mathematical discourse. Select and sequence some of the student solution strategies and ask a student from the selected groups to share with the class from:
- most accessible to least accessible solution strategies and representations;
- most common/frequent to least common/frequent strategies and representations; or,
- choose another approach to selecting and sequencing student work.
The tools and representations you might see students using to convince their peers and/or the teacher include:
- Concrete manipulatives such as connecting cubes, square tiles or relational rods to model the relative quantities organized like a graph.
- Use of grid paper to create graphical representations including bar models, line plots and pictographs.
Have students share their strategies and reasoning for how to represent the quantity of candy collected by each person. Ask them to convince you and their peers that their answer is correct by sharing mathematical models.
Discuss their strategies and elicit student thinking during your consolidation to build off of their current prior knowledge and understanding rather than “fixing” or “funnelling” student thinking to a strategy and/or model that does not connect to their strategy and/or approach.
Student Approaches
Student Approach #1: Concrete With Relational Rods
Student Approach #2: Graphing With a Line Plot
Student Approach #3: Bar Graph on Grid Paper
Next Moves
Reveal
Consider watching this video as a guide through the Consolidation Prompt portion of this task.
Consolidation
During the consolidation, you might consider exploring the relative quantities of candy based on the descriptors and their corresponding graphical representation. Some students might need a concrete tool such as square tiles to make sense of some of the more complex comparisons (i.e. 1 and 1/3 as great). The conversation should also highlight that this data is categorical and discrete and should therefore be represented using an appropriate graph (i.e. spaces between the bars) and a nominal scale on the x-axis.
You might consider using the following visuals to help guide the consolidation, or to help plan what models you might draw during the consolidation.
Reflect and Consolidation Prompts
Provide students an opportunity to reflect on their learning by offering these consolidation prompts to be completed independently.
Consolidation Prompt:
Lucia’s parents love candy just as much as the kids who attended the party. Her parents each grabbed some handfuls of candy throughout the party. Her Dad collected about one third the amount of the candy that Lucia collected, while her Mom collected about 5 times the amount of candy that her Dad collected.
Create a graphical or visual representation to compare the quantity of candy collected by Lucia and her parents.
About how much more candy did Lucia’s Mom collect than Lucia?
We suggest collecting this reflection as an additional opportunity to engage in the formative assessment process to inform next steps for individual students as well as how the whole class will proceed.
Resources & Downloads
Printable Lesson Plan PDF
Videos, Images & Media Files
Apple Keynote Presentation
Powerpoint Presentation
Printable Consolidation Prompts
Educator Discussion Area
Login/Join to access the entire Teacher Guide, downloadable slide decks and printable handouts for this lesson and all problem based units.
Explore Our 60+ Problem Based Units
This Make Math Moments Lesson was designed to spark curiosity for a multi-day unit of study with built in purposeful practice, number talks and extensions to elicit and emerge strategies and mathematical models.
Dig into our other units of study and view by concept continuum, grade or topic!
