CAN DRIVE
MULTIPLICATION
Real world multiplication lesson leveraging facts of 2, 5 and 10.
Intentionality
Spark Curiosity
Fuel Sensemaking
During Moves
Student Approaches
Next Moves
Consolidation
Reflect and Consolidation Prompts
Resources & Downloads
Educator Discussion Area
Intentionality & Unit Overview

Length of Unit: 5 Days
Access each lesson from this unit using the navigation links below
In this task, students will develop a deep understanding of 1-digit by 1-digit multiplication through the use of the array model. They will begin by leveraging their knowledge of multiplication facts of 2 to build fluency and confidence in solving multiplication problems. The array model will serve as a visual and hands-on tool to help students connect their prior knowledge of skip counting and repeated addition to the concept of multiplication.
Students will:
- Build arrays for multiplication problems, understanding how rows and columns represent factors and the product.
- Use the array model to model multiplication facts and explore patterns in products, particularly those involving 2.
- Begin to explore the distributive property, breaking up larger numbers into smaller, more manageable parts to simplify multiplication.
- Practice multiplication using hands-on tools and visual aids, gradually moving from concrete to abstract understanding.
Spark Curiosity
What Do You Notice? What Do You Wonder?
Show students this video:
Then, ask students:
What do you notice?
What do you wonder?

Give students 30 seconds (or more) to do a rapid write on a piece of paper.
Then, ask students to share with their neighbours for another 30 seconds.
Finally, allow students to share with the entire group. Be sure to write down these noticings and wonderings on the blackboard/whiteboard, chart paper, or some other means to ensure students know that their voice is acknowledged and appreciated.
Some of the noticing and wondering that may come up includes:
- I notice a table.
- I notice 8 cans.
- I wonder why they have cans.
- I notice there is room for more cans.
- I wonder what the cans are for.
- I wonder if there will be more cans.
At this point, you can answer any wonders that you can cross off the list right away. For example:
This class is collecting cans for a can drive.
A can drive is a community initiative where people collect canned food items, usually to donate to food banks or charitable organizations that help those in need. It’s a way to gather non-perishable food items for people who may be experiencing food insecurity.
In this scenario, the school is organizing a can drive, and the first class arranges their cans in a row of 8 on their table.
The array structure (1 x 8) helps visually organize the cans, making it easier to know the total number of cans. Since there are 8 spots, it’s a simple multiplication problem:
1 x 8 = 8 cans in a row.
Fuel Sense-making
Crafting A Productive Struggle: Prompt
Since you have already taken some time to set the context for this problem and student curiosity is already sparked, we have them in a perfect spot to help push their thinking further and fuel sense making.
Share the following silent animation.
Student prompt:
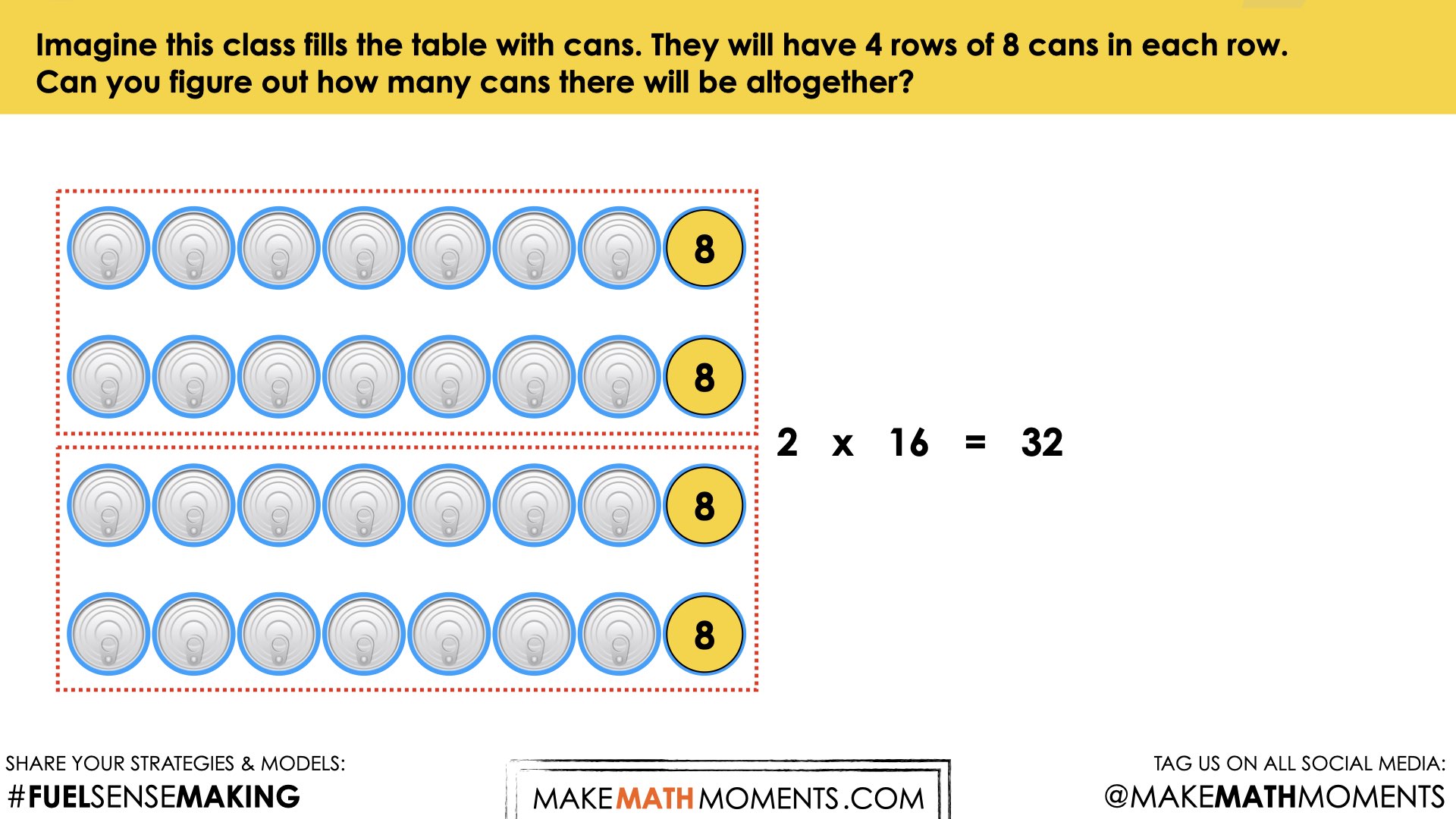
Imagine this class fills the table with cans. They will have 4 rows of 8 cans in each row.
Can you figure out how many cans there will be altogether?

Be sure to remind students that they are not to use a calculator to determine the number of cans as using that tool will rob them of this mathematical experience. Students should use a mathematical model in order to communicate their thinking.
During Moves
While Students Are Productively Struggling…
Monitor student thinking by circulating around the room and listening to the mathematical discourse. Select and sequence some of the student solution strategies and ask a student from the selected groups to share with the class from:
- most accessible to least accessible solution strategies and representations;
- most common/frequent to least common/frequent strategies and representations; or,
- choose another approach to selecting and sequencing student work.
The representations and strategies you might see students using to convince their peers and/or the teacher include:
- Concrete manipulatives to create the four rows of 8.
- A drawing to show the four rows of 8.
- Counting the cans individually.
- Skip counting.
- Using repeated addition.
- Using multiplicative thinking.
- Using a doubling strategy.
Have students share their strategies and reasoning for how to represent the number of cans in 4 rows. Ask them to convince you and their peers that their answer is correct by sharing mathematical models.
Discuss their strategies and elicit student thinking during your consolidation to build off of their current prior knowledge and understanding rather than “fixing” or “funnelling” student thinking to a strategy and/or model that does not connect to their strategy and/or approach.
Student Approaches
Student Approach #1: Concrete Array and Counting the Individual Cans
I made four rows of 8 cans out of the counters and then I counted them. I counted one at a time to get to 32 cans altogether.
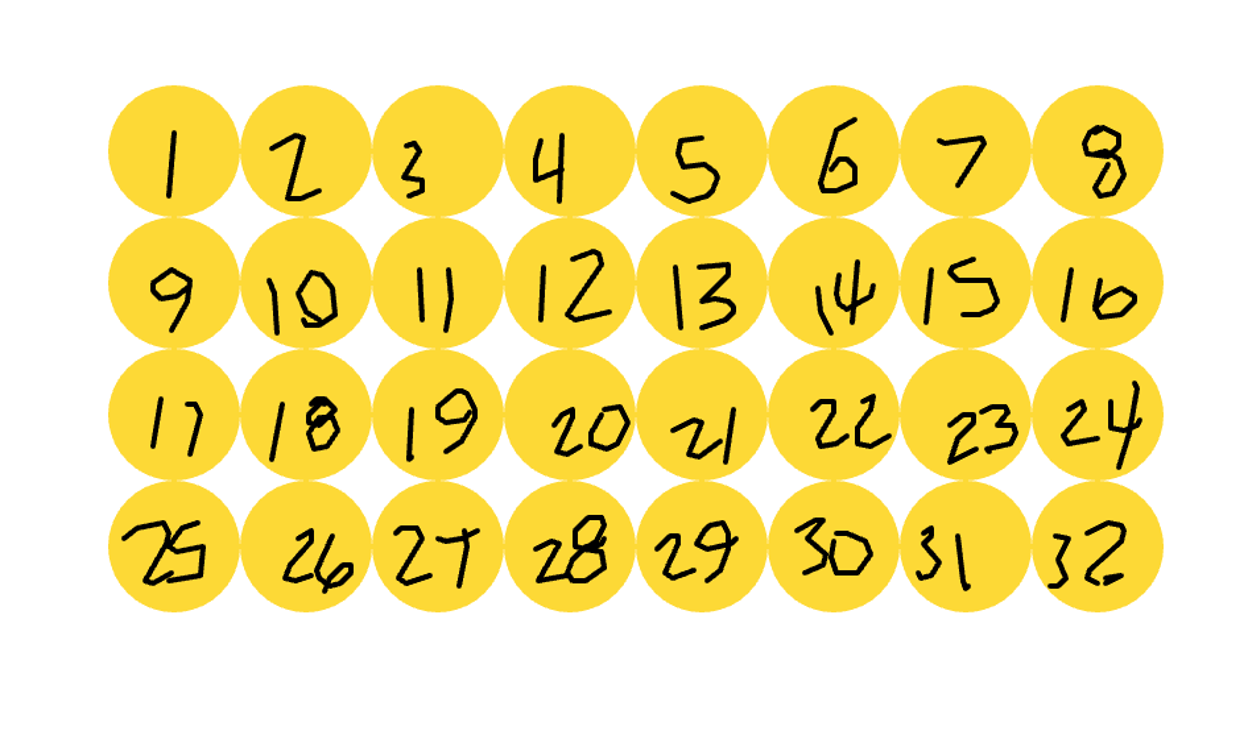
Student Approach #2: Pictorial Array and Skip Counting by 8
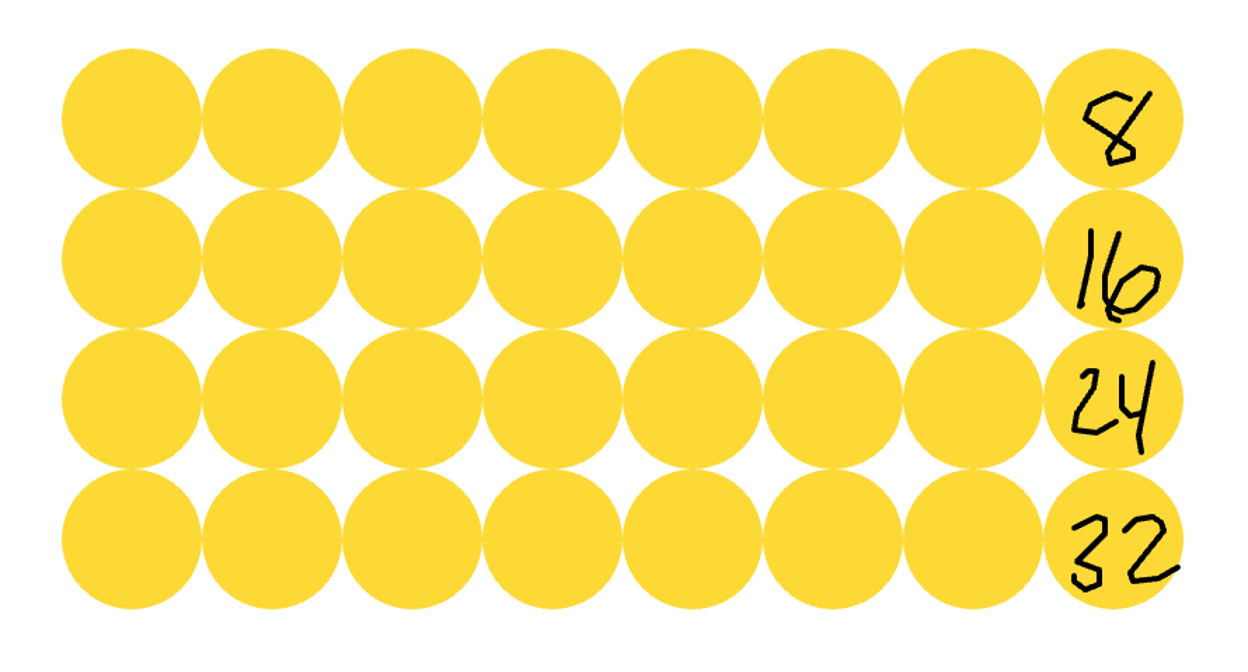
I drew the four rows, and then I counted by eight: 8, 16, 24, 32.
Student Approach #3: Pictorial Array Using Repeated Addition of 8
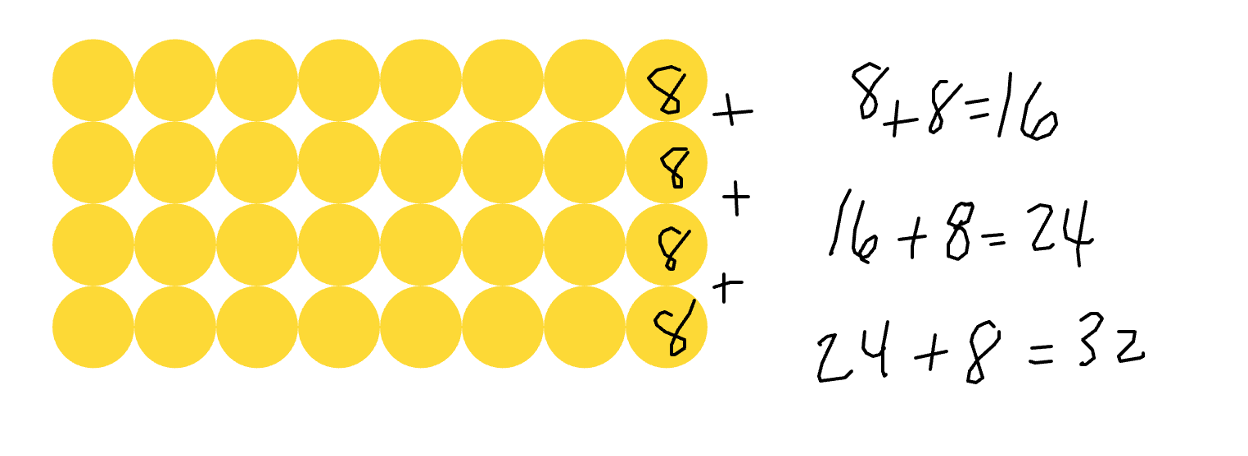
I drew the four rows of eight and then I added 8 + 8 + 8 + 8
Student Approach #4: Pictorial Array with Doubling

I know that 8 + 8 is 16, so then 16 + 16 = 32.
Next Moves
Reveal
Show students the following reveal video: Or consider sharing the final screenshots of the video: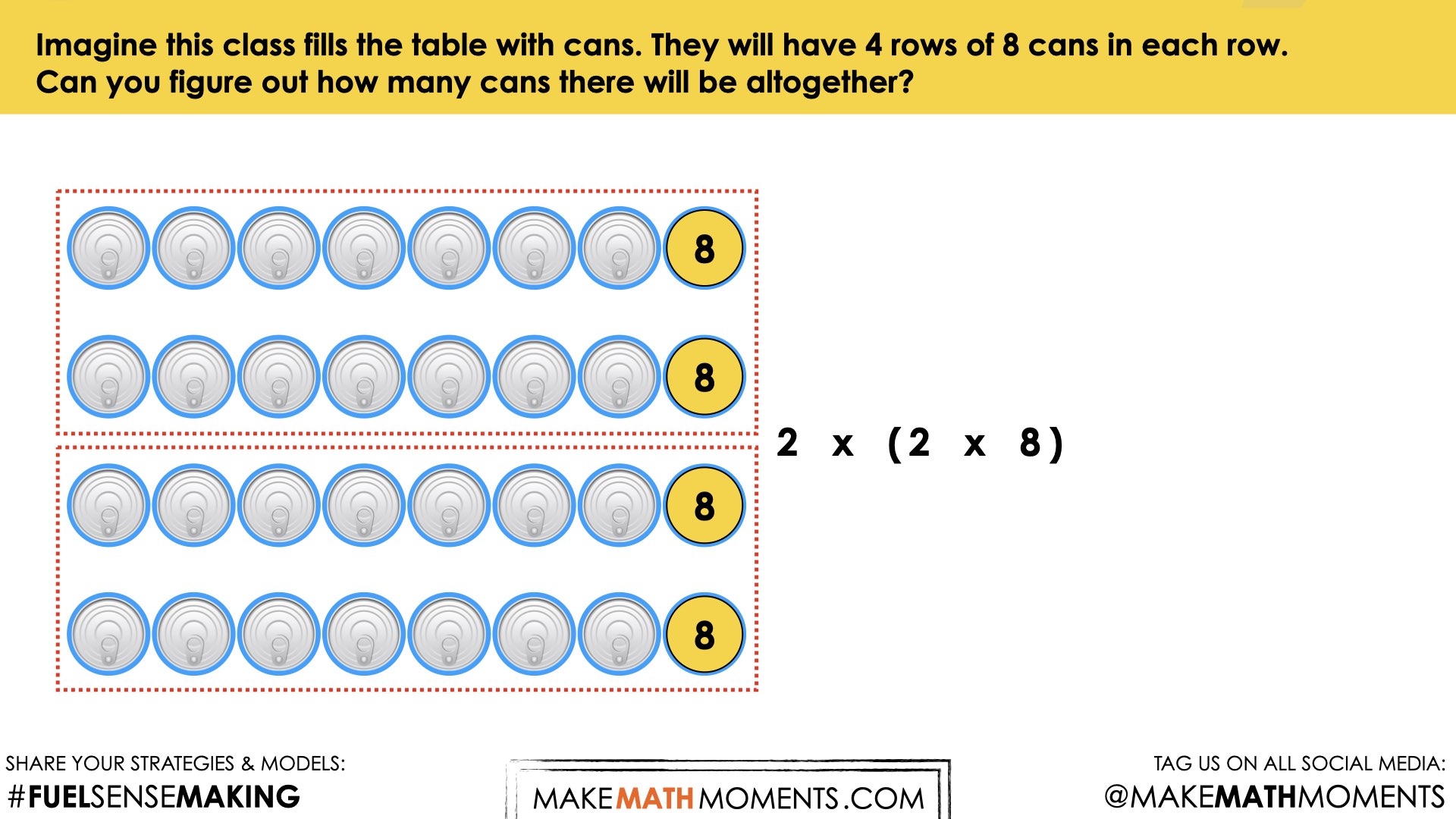
Consolidation
To help students understand 4 x 8 and its connection to multiplication, we can build on their initial approaches like skip counting or repeated addition.
Start by discussing how skip counting by 8’s (8, 16, 24, 32) shows they are essentially multiplying 4 groups of 8, but we want to emphasize that this is multiplication—not just counting.
Next, if a student uses repeated addition (8 + 8 + 8 + 8 = 32), guide them to recognize that multiplication is a more efficient way to represent repeated addition, especially when organizing it in an array model (4 rows of 8).
Finally, highlight how using the fact of 2 can simplify the process. By recognizing 2 x 8 = 16, students can double that result to find 2 x 16 = 32, making the problem more manageable. This approach shows how multiplying by 2 helps break larger problems into simpler parts and highlights the efficiency of multiplication.
During consolidation, connect these ideas, reinforcing that multiplication is about grouping equal sets, and using known facts (like 2) makes larger problems easier to solve.
Reflect and Consolidation Prompts
Provide students an opportunity to reflect on their learning by offering these consolidation prompts to be completed independently.
Consolidation Prompt #1:
🔵🔵🔵🔵🔵🔵
🔵🔵🔵🔵🔵🔵
🔵🔵🔵🔵🔵🔵
🔵🔵🔵🔵🔵🔵
Consider this array.
What multiplication sentence is represented by this array?
Now, think about how you can break apart this array to make the multiplication easier. Can you split the array into smaller sections? How would this help you solve the problem faster?
Explain your thinking and defend your strategy. What did you do, and why does it make sense?
We suggest collecting this reflection as an additional opportunity to engage in the formative assessment process to inform next steps for individual students as well as how the whole class will proceed.
Resources & Downloads
Printable Lesson Plan PDF
Videos, Images & Media Files
Apple Keynote Presentation
Powerpoint Presentation
Printable Consolidation Prompts
Educator Discussion Area
Login/Join to access the entire Teacher Guide, downloadable slide decks and printable handouts for this lesson and all problem based units.
Explore Our 60+ Problem Based Units
This Make Math Moments Lesson was designed to spark curiosity for a multi-day unit of study with built in purposeful practice, number talks and extensions to elicit and emerge strategies and mathematical models.
Dig into our other units of study and view by concept continuum, grade or topic!



