INVASIVE SPECIES [DAY 4]
EXPONENTIAL GROWTH & DECAY
Through the context of invasive species growth and the use of patterning, students will analyze the relationship between the size and unit of an exponent and the value of a power.
Intentionality
Math Talk
Visual Math Talk Prompt #1
Visual Math Talk Prompt #2
Visual Math Talk Prompt #3
Purposeful Practice
Resources & Downloads
Educator Discussion Area
Intentionality & Unit Overview

Length of Unit: 5 Days
Access each lesson from this unit using the navigation links below
Students will extend patterns with exponential growth and decay, make near and far predictions about the pattern, describe the pattern in words, and determine the general term.
Intentionality…
The purpose of the Day 4 activities is to reinforce key concepts from Day 3. Students will engage in a math talk, analyzing graphical representations of exponential patterns, and will have an opportunity to complete independent purposeful practice. The math talk and purposeful practice serve to develop a deeper understanding of the following big ideas:
- Patterns can be extended because they are repetitive by nature.
- Pattern rules are generalizations about a pattern, and they can be described in words.
- One common use of patterns is predicting future events.
- A pattern can be extended to make a prediction.
- For far predictions, calculations are required for efficiency.
- An expression that represents repeated multiplication of the same factor is called a power.
- Exponential growth is a pattern of data that shows greater increases with passing time.
- Exponential growth is a pattern that increases at a rate proportional to its current value.
- Exponential decay is a pattern that decreases at a rate proportional to its current value.
- Exponential growth is a pattern of data that shows greater increases with passing time.
- Exponential decay occurs when the base is greater than 0 and less than 1.
- In a power, the base number is defined as a number which is multiplied by itself.
- In a power, the exponent represents the number of times the base number is multiplied.
- Graphical representations of exponential growth appear as a curved line.
Math Talk
Share the following tables one at a time. Ask students to share what they know about the pattern. Develop a context that could be represented by this pattern. Describe the pattern rule in words. Collaboratively create an expression for each pattern by writing an expression. Use the expression to determine the value of the 5th term.
The string of exponential growth and decay patterns include:
y = 5x
y = 8 000(\(\frac{1}{10}\))x
y = 729(\(\frac{1}{3}\))x
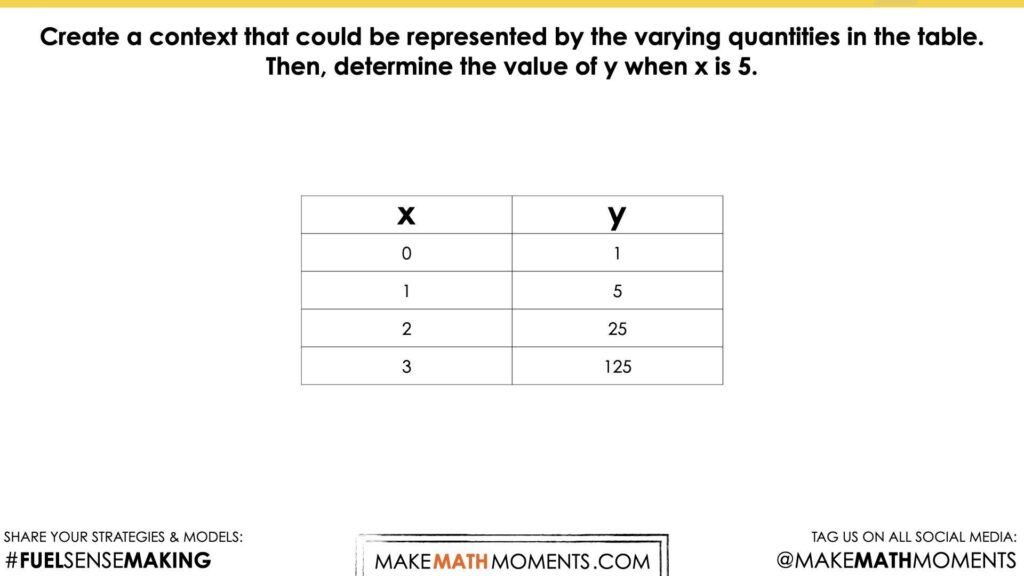
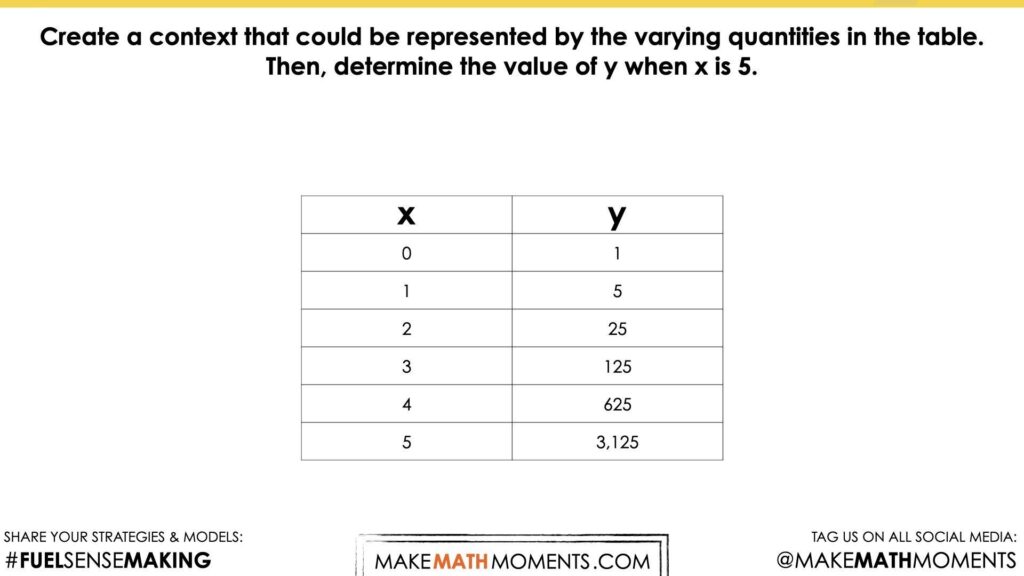
Visual Math Talk Prompt #1
Show students the following visual math talk prompt and be prepared to pause the video where indicated:
Students will be prompted with:
Create a context that could be represented by the varying quantities in the table.
Then, determine the value of y when x is 5.
Students may attempt to extend the table and identify the pattern relating the dependent variable, y, to the independent variable, x. In this case, as x increases by 1, y increases by a factor of 5.
Are students able to articulate how y changes relative to x?
Ask students how they might identify whether the pattern is increasing linearly or not.
What might we look for to determine whether y increases additively or multiplicatively?
The contexts students create may be related to plant growth since that was the context we shared this unit, while others might extend to human or animal population growth, disease, or even a “chain email” where people have to forward an email to 5 people, then they all have to forward the email to 5 more people, etc.
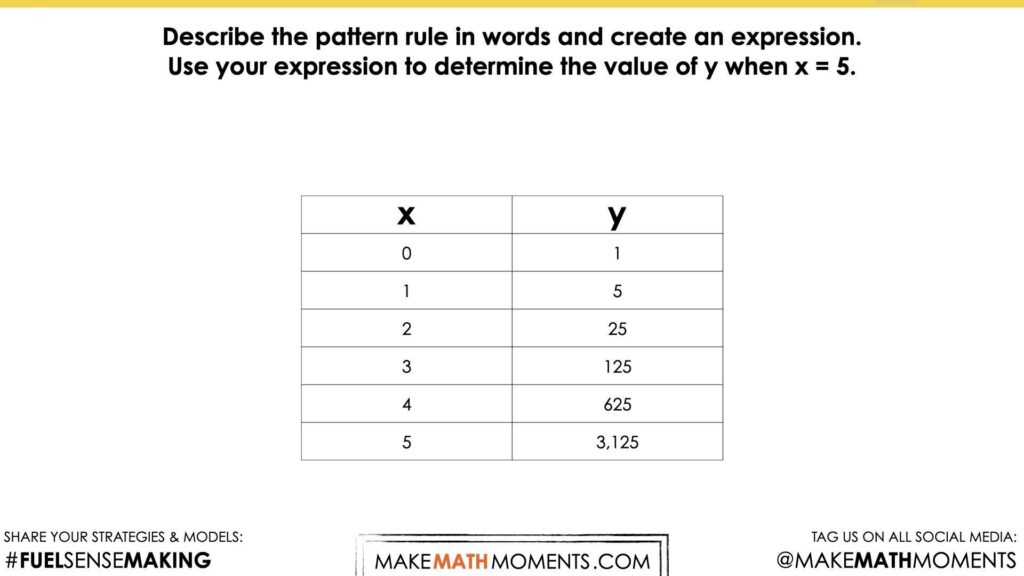
Prompt students with:
Describe the pattern rule in words and create an expression.
Use your expression to determine the value of y when x = 5.
While some students may struggle to describe the pattern rule in words and in particular with creating an expression, use this opportunity to explicitly highlight the verbal and algebraic pattern rules (i.e.: the expression and/or equation) to ensure they understand how this relationship is increasing.
Also be sure to note to students that this type of pattern has a name and it is an exponential relationship or exponential growth pattern.
Visual Math Talk Prompt #2
Login/Join to access the entire Teacher Guide, downloadable slide decks and printable handouts for this lesson and all problem based units.
Visual Math Talk Prompt #3
Login/Join to access the entire Teacher Guide, downloadable slide decks and printable handouts for this lesson and all problem based units.
Purposeful Practice
Login/Join to access the entire Teacher Guide, downloadable slide decks and printable handouts for this lesson and all problem based units.
Questions: Patterns with exponential growth and decay
Question #1:
Login/Join to access the entire Teacher Guide, downloadable slide decks and printable handouts for this lesson and all problem based units.
Question #2:
Login/Join to access the entire Teacher Guide, downloadable slide decks and printable handouts for this lesson and all problem based units.
Question #3:
Login/Join to access the entire Teacher Guide, downloadable slide decks and printable handouts for this lesson and all problem based units.
Question #4:
Login/Join to access the entire Teacher Guide, downloadable slide decks and printable handouts for this lesson and all problem based units.
Question #5:
Login/Join to access the entire Teacher Guide, downloadable slide decks and printable handouts for this lesson and all problem based units.
Resources & Downloads
Login/Join to access the entire Teacher Guide, downloadable slide decks and printable handouts for this lesson and all problem based units.
Printable Lesson Plan PDF
Educator Discussion Area
Login/Join to access the entire Teacher Guide, downloadable slide decks and printable handouts for this lesson and all problem based units.
Explore Our 60+ Problem Based Units
This Make Math Moments Lesson was designed to spark curiosity for a multi-day unit of study with built in purposeful practice, number talks and extensions to elicit and emerge strategies and mathematical models.
Dig into our other units of study and view by concept continuum, grade or topic!